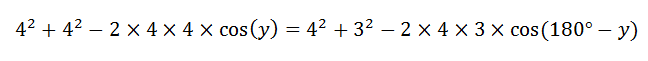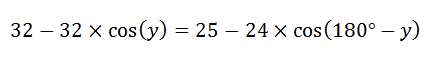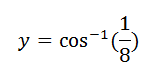Tag: math (page 5 of 10)

April 25, 2012 / 0 Comments

Converting degrees to radians

One of my students came up (with some help) this procedure for converting between degrees and radians.

Memorize the fact that 60° is π/3 and that 30° is π/6.
Note that 10° is therefore π/18 and that similarly 1° is π/180.
You can then take any degree measure and convert it by converting the number of degrees into sums of degrees where you know the conversions. For example, 70° is equal to 60° + 10° = π/3 + π/18 = 6π/18 + π/18 = 7π/18.

Obviously this procedure is not by any means the most efficient way to convert between radians and degrees. Although I showed a much more efficient algorithm for converting between degrees and radians, it didn’t make sense for this student, and so he and I came up with this procedure (which I drew out of him by asking him questions about the angles), which he does understand.

In general, I’d prefer students use inefficient techniques that they understand completely than highly efficient techniques that they do not understand. Hopefully this student will continue to work on his procedure to make it more efficient as he has to use it over and over again, but if not, at least he will be thinking with something that makes more sense in his head.

March 20, 2012 / 4 Comments

Math in the real world: Gardening

My uncle called me today, and asked me a math question. Normally, I get called and asked technology related questions, but occasionally people remember that I have a mathematics background and call me in to assist.

My aunt wants to build a raised garden bed with a very particular shape. My uncle has been tasked with building it. She wants 3 of the sides of the shape to be 4 feet long, and the 4th side to be three feet long, and the whole shape should form a trapezoid (with a line of symmetry down the middle of the trapezoid). It took a little bit of chatting on the phone to get this to be clear, and I can see how being able to send each other pictures would have been really useful. To be able to build this shape as accurately as he would like, he needs to know all of the angles of the shape, so he can cut the pieces of the wood with the angles in the right position using a miter saw.

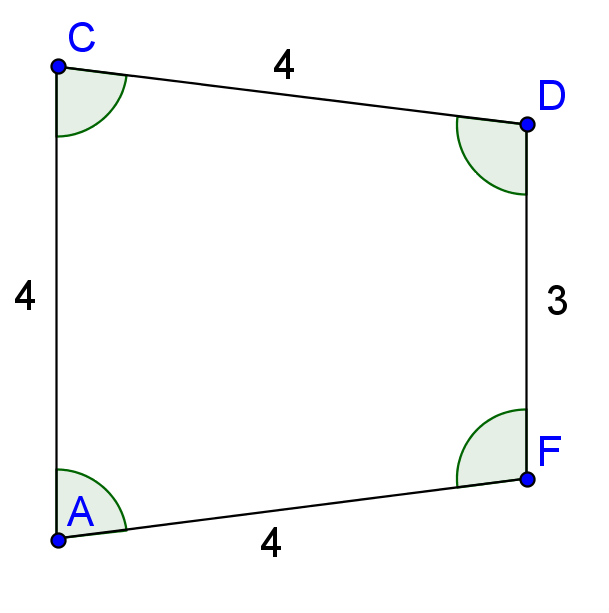
Trapezoid garden bed

I looked at the shape and decided that the fastest solution would be to build the shape in Geogebra, and measure the angles, which resulted in this.

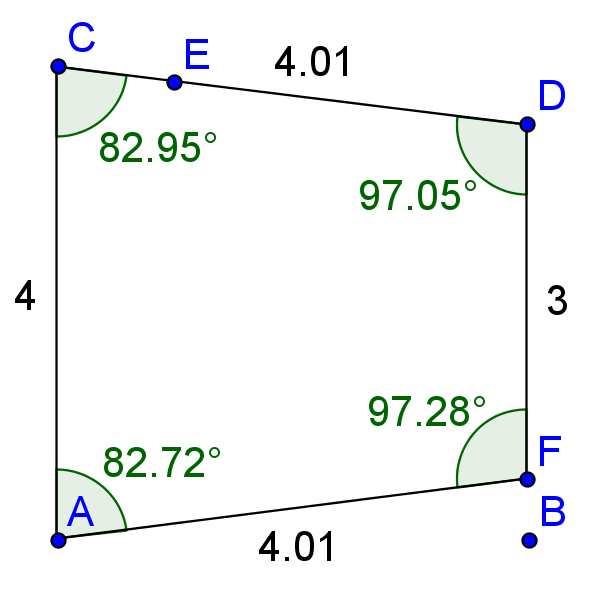
Not the exact solution, but close enough that my uncle would be able to use the miter saw (which has a maximum accuracy of 1 degree, according to my uncle) and cut the wood for his shape. It took me about 3 or 4 minutes to draw the shape in Geogebra and measure the angles.

After my phone call with my uncle was over, I decided that I should double check this solution though, and verify that I knew how to solve it.

I drew an imaginary line across the shape, and labelled that side x. This allowed me to create a pair of equations using the Cosine law, and I ended up with the following equation to solve:

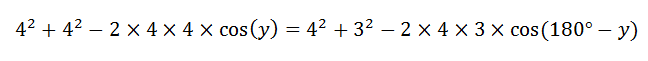
First equation

which simplifies to:

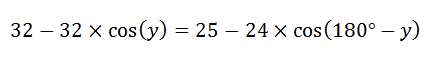
Second equation

and finally leads to this calculation:

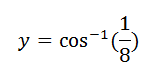
Third equation

On my calculator, that leads to a value of the smaller angle of about 82.8° and a larger angle of 97.2°, which means that my diagram that I drew for my uncle is fairly close. Wanting to be sure that my answer was correct, I also checked it using Wolfram Alpha, and on my graphing calculator.

After I told my uncle the solution, he told me that my aunt had suggested drawing the diagram carefully on a piece of paper and measuring the angles with a protractor, but he had complained that solution wasn’t "mathematical enough." Of course, this leads to a discussion of what it means to do mathematics, anyway.

Does it matter which way I solve this problem for my uncle? Which of these techniques would you classify as "mathematics"? All of them? None of them?

March 13, 2012 / 0 Comments

Articles I’ve written on Math Education

Here is a list of some of the articles I’ve written on Math Education

On Mathematics education reform:

On the use of technology in mathematics education:

Exploring matrices

I introduced matrices to my students last week. Together, we worked out the algebra necessary to find the inverse of a 2 by 2 matrix, and developed the idea of the determinant of the matrix. The algebra was hard for my students, and we focused on looking for patterns. I showed students how a matrix can be applied to solving simultaneous equations, so they understood that there is some context for matrices. Next week, I intend to show some of the applications of matrices to game theory, and have students explore the consequences of this application.

Today, I talked about the inverse of a 3 by 3 matrix, which was easily found on a calculator. However, for good or for ill, students are expected to know how to find 3 by 3 matrix inverses "by hand" in preparation for their IB Math SL exam. So we needed a way to understand how the inverse of a 3 by 3 matrix is formed. I showed students that one can construct 2 by 2 matrices within the 3 by 3 matrix, and that the determinants of these 2 by 2 matrices "magically" appear in the inverse of the 3 by 3 matrix. The proof of this (for 11th grade students) is hard.

So instead of going through the proof, I decided that students should explore the relationships between the positions of the 2 by 2 embedded matrices, and where their determinants appear in the 3 by 3 matrix. I don’t myself have an intuitive sense of exactly where these determinants will show up, but I know there is a pattern, and that my students will find it.

What was fascinating to me is the different ways students represented the notation necessary to show these patterns.

Examples of student notation. Click to enlarge.

Each student came up with their own notation to represent the patterns they were finding. They realized (some of them with some guidance) that it was pretty critical to include the location of the 2 by 2 matrix in their notation, to make it easier to find patterns. The actual notation they use doesn’t matter to me, as if they do continue with matrices, they’ll learn the appropriate notation later. What was critical for me was that they could come to some understanding of how to find the inverse of a 3 by 3 matrix.

What I found most interesting about this activity is that there is room for exploration in learning matrices, which suggests to me that it is very likely that any mathematical topic has some opportunity for exploration.

February 20, 2012 / 3 Comments

Taking advantage of the mobile nature of a mobile device

An iPhone (or any other smart phone / tablet) is a mobile computing device. Applications that are designed for a mobile computing device should take advantage of the mobile nature of the device. Too many educational (cr)apps are designed simply as better flash card systems. They rarely take advantage of the most important affordances of the mobile devices they are on, and are easily replicated without using the technology.

Not only can you take pictures and videos of the world wherever you are able to travel, with a little bit of hardware, you can turn your iPhone into a mobile microscope, allowing you to view the microscopic world, which combines the mobile nature of the smart phone with its computing power. Your GPS in the iPhone allows you to participate in Geocaching.

Have an idea on the go? Use your smart phone to record a note about the idea, or a create a podcast on the fly. You can use the Internet capability of your smart phone to collaboratively keep track of data (or anecdotal observations) when out in the field. Heading out bird watching? Keep track of your GPS, a photo of the bird, and any other anecdotal evidence you need with that one device in your pocket.

The point is, try and find the educational uses of Smart phones which actually take full advantage of the capabilities of the phone, rather than limiting kids to using the phone as an extremely small computer screen.

January 24, 2012 / 7 Comments

Do iPads improve mathematics instruction? Maybe

Student using iPad
(Image source: MindShift blog)

Stephen Downes just shared this study suggesting that students see a 20% improvement in their test scores on their state exam after using an iPad loaded with HMH Fuse.

I am a supporter for using technology in mathematics education, but it’s probably worth examining these results closer. Here are some quotes from the study itself, and my unpacking of what this means for the reliability of this study.

“Earhart has been a school eager to employ new technology in the classroom…” (p5):
This suggests a selection bias. Further, it also suggests that this program has been attempted at one school, or that all that has been shared with us are the results from one school. Were there other schools that had an opportunity to pilot this program which have not been shared in this study?
“Coleman approached his teachers about this opportunity and two teachers, Jackie Davis and Dan Sbur, were ultimately chosen to take part in the study.” (p5) :
This suggests that the process for choosing the teachers was anything but random. The study makes careful mention that the students were carefully chosen, but underplays how teachers were selected.
“…this meant more work and time required by the teachers…” (p5) “Like any new technology, there was a slight learning curve with adopting a tablet in the classroom. “In the beginning of the year I tried a little bit of everything, trying to find out what was best for my class and for me,” recalls Jackie Davis. Dan Sbur also found that “Over time, it became easier to use and I could use it more in my class as I became comfortable with the device and app.” (p6) :
The teachers who were chosen (or volunteered?) had to work harder to implement this program. This suggests that at least part of the effect on their test scores could be attributed to the efforts their teachers put in.
“…This meant that students were allowed to take the devices home and “customize them,” adding their own music, videos, and additional apps. This approach also allowed students to have 24/7 access to the HMH Fuse: Algebra I program.” (p5) :
So now, are we measuring the effectiveness of the program, or the effectiveness of time spent learning math? Students who spend much more time working on math are obviously going to see an increase in their test scores.
“As one would expect, those students who were randomly selected to be part of the HMH Fuse study were very excited – as were their parents. In fact, Coleman quickly found that one benefit of the HMH Fuse: Algebra I app was enabling parents to provide more support to their children: “Parents could watch the videos or review problems with their children to help them if they did not understand.”” (p6) :
Clearly parental involvement makes a difference in a student’s education, and if this app helps parents be more involved, that’s excellent. If this program wasn’t considered so innovative, and new, would parents be as involved? In other words, if we standardized this program, would parents get excited by it?
“In addition, Mr. Davis found students took the initiative to use HMH Fuse: Algebra I to check their work during class, freeing him up to do more one-on-one work with struggling students in need of individual attention. In this regard, the HMH Fuse app essentially enabled a “flipped classroom” model in which students learned and worked independently at home, and then came to class ready to do problems and practice what they had learned (see Bergmann & Sams, 2011). This “flipped classroom” dynamic gave both Mr. Davis and Mr. Sbur the ability to provide personalized instruction to many students during the normal school day.” (p6) :
If the HMH Fuse app allows students to work in a more self-directed way, that’s a good thing. If their teachers are changing their pedagogical approaches to suit the affordances of the device, that’s probably a good thing too. So one wonders how much of the learning effect was due to this personalized attention. Did the two teachers in this study also find ways to personalize and give individual attention to their students in their other non-iPad classes?

One thing not at all discussed in this study is what they hope to accomplish by improving mathematics instruction. Test scores are one measure we have for mathematical ability, but they are not the only measure. Did this program give students additional time to work on improving their mathematical reasoning and their problem formulating & solving skills? Hopefully the authors of this paper will submit it for formal review so that any of the issues that I’ve addressed can be peer reviewed.

November 28, 2011 / 0 Comments

Experiments in assessment

Here a few experiments in assessment I’m considering for next year.

Compare the results between an oral assessment (as in, find out what they can tell me they know verbally) and a traditional test. . Question: How much of a difference does the mode of assessment make?
Compare the results between a 10 minute quiz and a full length test. Question: Do I find out significantly more with a longer assessment?
Give my students an assessment where I only give them written feedback and no numbers or check marks. Compare this with an assessment where I only give check marks, and another where I only give numeric feedback. Question: Are the numbers and check marks necessary?

What other experiments would you suggest that I try?

November 25, 2011 / 0 Comments

Mathematics in the real world: World Statistics

This is another post in my series on mathematics in the real world.

Thanks to a colleague of mine, I rediscovered the Google Public Data explorer. Within 10 minutes, I had constructed the above graph, which shows adolescent fertility rate for 15 to 19 year olds, versus life expectancy, measured against (look at the colors) average income for all of the countries in the world. If you click play, you can see a happy trend; life expectancy is increasing across the world for almost all countries, and the fertility rate is also decreasing.

This type of graph also lends itself well to questions from your students. For example, they may ask why so many teenagers have babies in some countries. They may also why there is a relationship (and from the above graph, it looks like the relationship is reasonably strong), between births from teenage moms, and life expectancy. They may also ask about trend itself, and why that is happening. Further, they may ask, how strong is this relationship? They may also confuse correlation with causation, which in itself can lead to an interesting conversation.

A natural extension of an activity related to this graph would be to have students construct their own graphs, perhaps even collecting their own data. What kind of social data do you think would interest your students?

November 21, 2011 / 2 Comments

What is math?

This image is an attempt to capture the important stages of doing mathematics. As pointed by other people, mathematics is not a linear process, which I am attempting to share via this image. I see analytical reasoning, flashes of insight, and exploratory calculations as the glue that holds these stages of mathematical thinking together.

The stages to doing math

How do you see the process of "doing math"? Is it possible that what sets mathematics apart from other disciplines is the formalism, and the calculations involved? How does this process compare to other things that we do in life?

November 18, 2011 / 2 Comments

Why math instruction is unnecessary

This TED talk by John Bennett raises an important question; why do we teach middle school and high school math?

I don’t know if using "puzzles" is a scalable solution for the problems in mathematics instruction in middle schools and high schools. It would probably work for many math teachers, but wouldn’t necessarily work for all math teachers. Puzzles and games are good for teaching analytical skills, provided you have someone around who models the use of analytical skills during the game. I’ve noticed, over many, many years of playing games, that many of my friends do not use much deductive reasoning during games. What I would support is much more use of puzzles and games during mathematics class than what is currently considered acceptable practice.

John’s argument that middle school and high school mathematics is unnecessary should actually be restated: our current middle school and high school mathematics curriculum is unnecessary. John is essentially arguing for a different curriculum, rather than discarding the practice of developing mathematical reasoning in students.

I think we need a variety of approaches. What we are doing right now works when students have a strong mathematics instructor, but isn’t working for every student. Instead of assuming that there is one solution to the mathematics education "problem", we should recognize that there are a variety of solutions. What works for John Bennett may not work for every mathematics teacher. I’d like to see these different solutions compete more with each other, and be able to do more research on the effectiveness of each of these approaches. We definitely need more flexibility in mathematics instruction, especially with regard to the curriculum outcomes.

I think we should be focusing less on curriculum outcomes, and more on the holistic goals of a mathematics education. I don’t think it matters if every student learns about the quadratic formula (for example), but all students would benefit from learning deductive and inductive reasoning, pattern finding, modelling of data, and problem formulation. Curriculum should be a vehicle for these goals, rather than the goal itself.