Tag: The Reflective Educator (page 11 of 43)

July 16, 2013 / 6 Comments

Reflection on How to Learn Math: User interface matters

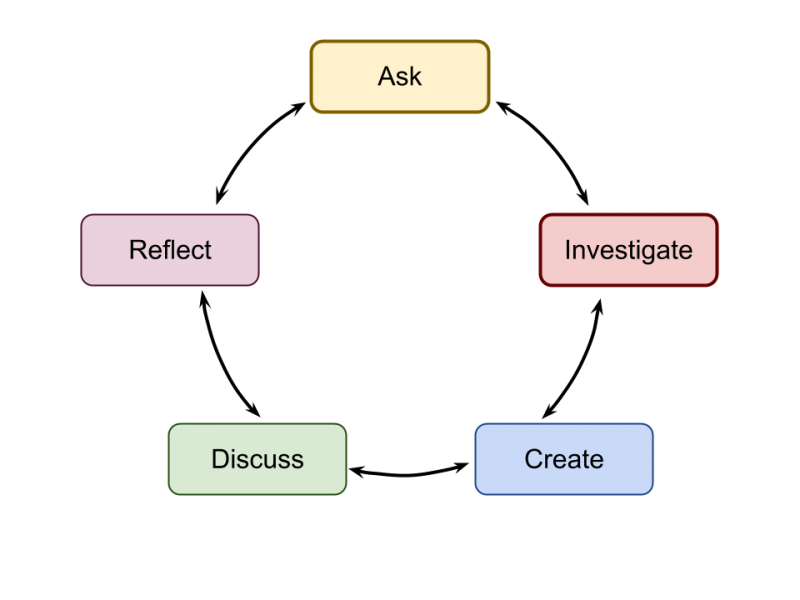
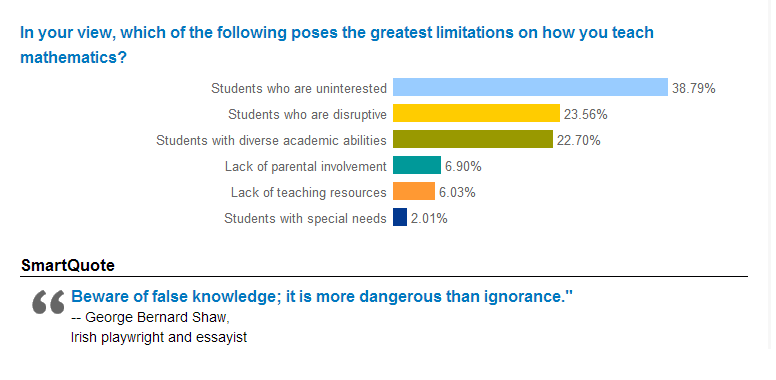
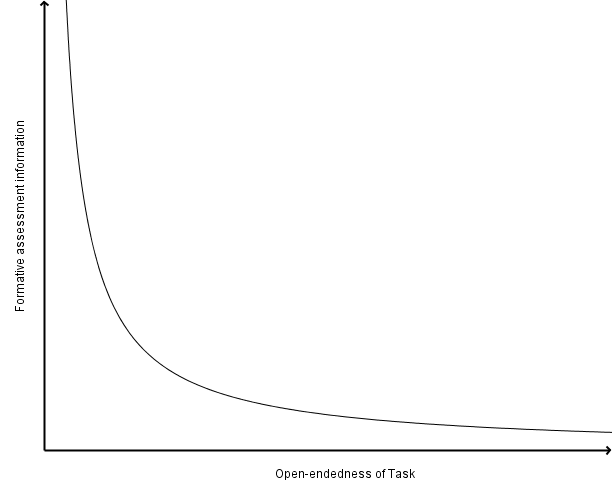
I’m participating in Dr. Jo Boaler’s course "How to learn mathematics" which started two days ago. Here are my observations so far:

I like the structure of the ideas Dr. Boaler has presented so far. The "quizzes" we have done so far seem not to have right answers, and are more designed to make us think. The videos are short and engaging and easy to follow.
The premise of the course is excellent, and I think that this kind of course is best held in a discussion style, with some ideas being seeded by the instructor, which looks like the purpose so far of the course.
I read every single introduction people posted, and I was very impressed with people’s willingness to share that they have had poor experiences in math. I know that happens quite a bit (almost everyone I meet tells me they were terrible in math after I tell them what I do), but not so often in print, and I suspect not so often on the first day of a course. This almost certainly has something to do with the way Dr. Boaler framed the course which has clearly made people willing to start the course by candidly sharing their experiences.
Unfortunately, the user interface for discussion is awful, which I know has nothing to do with Dr. Boaler, since she is very likely constrained in what platform she uses (given that she works at Stanford). This is also an issue that I brought up with respect to Dr. Keith Devlin’s course on Mathematical Reasoning.

Once I participate in a conversation, I can see no way of finding out if anyone has responded to my conversation without looking up the conversation in the long, long, long list of other conversations that have happened. I have no "home base" with which to find conversations for later. I actually used CTRL + F to search for my name after loading the very long welcome thread to find my name! My first recommendation for improving this is that the designers of Stanford’s course software should look at other forum software, much of which has evolved over at least a decade of use, and not try to recreate a new user interface for a standard forum discussion. My next recommendation is to offer a way for users to see, in one place, who has responded to something they have posted, and to be able to choose to receive notifications when someone responds. The discussion space should be more like Facebook, and less like Moodle.

Another issue I have noticed is that I seem to have to scroll through the conversations and load them as I scroll. This means that if I am interested in finding an older conversation, I may potentially have to spend many minutes scrolling through unwanted conversations looking for the one I want.

This issue around the usability of the discussion space is an important one, but this course is very interesting to me, and I intend to work with the discussion space as offered.

July 14, 2013 / 8 Comments

What evidence convinces teachers to change practices?

Research, by itself, rarely changes teacher practices. Presentations on why their practices should change rarely change teacher practices. Attending conferences rarely changes teacher practices (a teacher may adopt a few new things from a conference, but how often has a teacher come back from a conference and begun to teach in a completely new way?).

What does change teacher practice?

Eric Mazur, in his lecture "Confessions of a Converted Lecturer", recounts how standard measures told him his teaching style was sufficient, but when he applied a different measure, he was quite surprised to discover that his teaching had nearly no effect on his students’ conceptual learning of physics. The evidence that convinced Eric that his teaching needed improvement was the results of investigating his own teaching using a different tool, the force concept inventory. The key here is investigating his own teaching, not necessarily the tool he used.

In an essay titled, "How One Tutoring Experience Changed My Teaching", Sara Whitestone recounts how she discovered that the writer’s voice in their writing matters, and how she had to do more to help her students develop their own voices, rather than adopting the writing voice of their teacher. The tutoring experience is not what changed her teaching, it was her reflection on that tutoring experience that changed it, but the experience acted as a catalyst for this reflection.

When I asked the question, what evidence shifts teacher practices, on Twitter, I had a few responses, which could be summed up with these two tweets.

@geonz @davidwees Agreed. If anything persuades, it’s experience. Few truly change practices based on argument or fact #mathchat #edchat

— Ben Orlin (@benorlin) July 12, 2013

Why do teachers often ignore evidence? It is probably because the evidence they are presented is not grounded in their own experiences, but in narratives of experiences other people are describing. In other words, the way they are presented with the evidence is not supported by their experiences, and so they do not learn from it.

It seems therefore, that if we want teachers to change practices, one method which may work is to ask them investigate for themselves what their practices are, and participate in an inquiry into their own teaching practices.

Ask, Investigate, Create, Discuss, Reflect
(Adapted from this)

What are some ways that you know are effective in promoting teacher growth and change of practices?

July 9, 2013 / 17 Comments

Ambiguity in mathematical notation

I’m reading Dylan Wiliam’s "Embedded Formative Assessment" book (which I highly recommend) and this paragraph jumped out at me:

"To illustrate this, I often ask teachers to write 4x and 4½. I then ask them what the mathematical operation is between the 4 and the x, which most realize is multiplication. I then ask what the mathematical operation is between the 4 and the ½, which is, of course, addition. I then ask whether any of them had previously noticed this inconsistency in mathematical notation — that when numbers are next to each other, sometimes it means multiply, sometimes it means add, some times it means something completely different, as when we write a two-digit number like 43. Most teachers have never noticed this inconsistency, which presumably is how they were able to be successful at school. The student who worries about this and asks the teacher why mathematical notation is inconsistent in this regard may be told not to ask stupid questions, even though this is a rather intelligent question and displays exactly the kind of curiousity that might be useful for a mathematician — but he has to get through school first!" ~ Dylan Wiliam, Embedded Formative Assessment, 2011, p53

Mathematical notation has been developing since the introduction of writing and has largely grown organically with new notation added as it is needed. In fact, if a mathematical concept is developed in different cultures, it is entirely likely that each culture will develop its own mathematical notation to describe the concept, and these mathematical notations inevitably end up competing with each other, sometimes for centuries.

This observation by Dylan Wiliam suggests to me that difficulties in mathematics for some students are almost certainly related to the notation that we use to represent it (especially in classrooms where mathematics is largely presented to students in completed form, rather than being constructed with students), and that people who end up good at math in school may be good at being able to switch meaning based on context.

Can you think of any other examples of mathematical notation which are potentially inconsistent with other mathematical notation? I’ll add one to get the list going: which is clearly inconsistent with algebraic notation, and potentially with fractions too.

July 5, 2013 / 4 Comments

Students who are uninterested in math

Results of the NCTM survey on why math is hard to teach

Source: NCTM Smartbrief

It seems to me that "students who are uninterested" is a problem of pedagogy. If that is what is holding students back from learning mathematics, then you should make your lessons more interesting. "Students who are disruptive" seems like another way of phrasing the first problem, but having worked in a challenging school myself, I do remember students who were challenging no matter how much I stood on my head to make my lesson interesting. That being said, both of these challenges are significantly less with good teaching. Students who are uninterested in what you teach are a sign that you should change your approach.

"Students with diverse academic abilities" is a serious problem, but it is (at least in part, see below) solvable. The basic trick is this, don’t teach everyone the same thing at exactly the same time. I would approach this particular issue with low-entry / high ceiling problem solving activities in small groups and then I workshop solutions with individual groups as I move around the classroom.

"Lack of a parental involvement" is definitely a huge issue. I think if you have interesting lessons and develop positive relationships with your students though you can mostly counteract the effects of parental apathy. My objective here is to set high standards for my students and their relationship with math like what I have for my own son.

"Lack of teaching resources." Uh… Have you heard of the Internet? Being a part of the Math Twitter Blogosphere means that lack of resources is never a problem. In fact, more resources than I can possibly use is more frequently the problem. Edit: It occurred to me that this may mean lack of physical resources, like pencils and paper, etc… in which case someone, somewhere, needs to rethink the priorities for their schools. Teachers and students should not lack for basic supplies.

For me the "students with special needs" problem can partially be addressed with using a problem solving approach with media that asks questions (like what Dan Meyer is curating with 101qs.com) for students for whom literacy is their barrier to mathematics. However, students with dyscalculia or who are many, many grade levels behind in their understanding of mathematics probably need more support. Having worked in a school that had minimal support for students with special needs many years ago, I definitely empathize with people who see this as a problem.

The thing is about all of these responses is that not one of them is how I would answer this problem. For me, the things that I feel impose the greatest limitations on how I would teach (and most importantly, what I would teach) are the standards we are assigned to teach and the way students will be eventually externally assessed on those standards. I can certainly still teach in a creative way given these limitations, but they definitely place limitations on how I teach.

July 5, 2013 / 0 Comments

Designing open-ended tasks – Part 1

Designing an interesting and open-ended task is relatively easy. The challenging part comes when you attempt to use the task and learn something as a teacher about what your students understand.

Graph of inverse relationship between open-ended tasks and formative assessment tasks
This graph represents the main issue that comes up when designing open-ended tasks for students to use; the more open-ended a task is, the less information you are likely to be able to gain from using the task. You can gain insight into what your students are thinking when they are working on an open-ended task, but chances are much greater that they have a much wider variety of insights and misconceptions that will come up during their time working on the task.

If we define "formative assessment" as "any tool used by teachers to gain insight into their student’s thinking and use that information for future planning of teaching and learning activities," then the open nature of these tasks helps with gaining insight into student thinking, but it makes planning future activities more challenging since we could potentially end up with much more information about what each student knows how to do, but potentially less information about what each student does not know how to do.

The very task that gives students the freedom to try many different potentially successful mathematics techniques to solve the problem unfortunately also limits how much of what we know of those paths that students chose not to follow. Did a student not use an algebraic approach because they don’t know algebra? Or did they use a non-algebraic approach because they didn’t think of an algebraic technique? Did they use a table to solve the problem because they love using tables? We have no time-efficient way of answering these questions.

One possible solution is to make sure that each time students work on a task, they have the opportunity to share their different solutions with each other. This way students who tend to use graphs to solve problems will see algebraic solutions to those same problems. Students who generally solve a problem using a table of values will see how other students used a diagram instead. By sharing different solutions created from the students amongst your class, students may be able to add to their own toolbox of methods they can use to approach problems.

The problem with this last solution is that this only works when the students are working on essentially the same problem; it will fail to work when the task is so open-ended that students have sufficient influence over the questions they ask. Maybe there are some other solutions to this problem?

In a future post, I will take a task and create different versions of it, sliding along the scale of open-endedness, and hopefully this will lead to some insights as to the challenge involved in open-ended tasks.

July 4, 2013 / 0 Comments

130 years of climate change data

Daniel Crawford is working on an interesting problem; how can we represent data about climate change in other ways. Each note he plays represents the average temperature for a year, with higher pitched notes representing higher temperatures. While I wouldn’t call this piece very musical, it is a very interesting and useful way to represent data about average temperatures.

Once people understand that our world is getting warmer, and significantly so, the next step is to wonder why. I’m interested to see what it would sound like if they overlaid these graphs with graphs of the average parts per million of various gases known to be associated with the greenhouse effect, and played by different instruments.

July 2, 2013 / 0 Comments

Drawing The Koch Snowflake with Blockly

Blockly Koch Snowflake

Google released Blockly, an open-source programming language accessible through the browser about a year ago, and very recently, they released a version of it that emulates the functionality of the Logo programming language. To test out how powerful the language is, I created a program to draw the Koch snowflake.

I recommend trying this out yourself (It works best in Google Chrome and Firefox, and I suspect it probably works fine in Safari. I haven’t tested Internet Explorer) and then thinking about a way you can integrate it into a mathematics lesson (or ten).

June 24, 2013 / 0 Comments

[Bad math] Polling

Poor polling practice

I hope no one takes the results of this poll by the Conservative party very seriously. Before they even collect results, I know that those results will have significant bias.

They promoted their own political party in large bold font just before their survey question. This is equivalent to saying "Chocolate is AWESOME!! Do you like chocolate, yes or no?"
They are relying on people to take the time to respond to the survey themselves, which suggests that they will end up with a small group of people who are die-hard opponents of the Conservative party, and probably a larger group of Conservative party supporters. Who else would bother voting in this poll?

Note: If you were to substitute any other political party into this poll or a similarly run one, it would be just as invalid.

June 22, 2013 / 2 Comments

Lego and Minecraft in Math

This article originally appeared in my school’s monthly magazine, and was co-written with Chelsea Todd, a 4th grade teacher at my school. Note that the lesson idea presented below is Chelsea’s idea.

The 4th grade math classes were recently completely engaged with a challenging but slightly unusual mathematical task. Students had been studying area and perimeter with the guiding question, “How do area and perimeter help us to construct things?”. For their summative project, students designed and constructed a model building design, starting first with the architectural blueprints and completing their project with the structure. They had the choice to build their world virtually using Minecraft or to use Lego blocks. During the two classes, it looked like every student was engaged in the task for the entire class time, and in fact sometimes their concentration on their work during the task made it difficult to interrupt them to ask questions!

In the first class, students designed blueprints on graph paper, being sure to include accurate labels of the values and calculations of each shape’s area and perimeter. They spent the entire time busy at work, trying to create complicated designs, knowing that they would be asked to recreate these designs the following day. They used their knowledge of area and perimeter to accurately label their work, often re-checking their values to ensure that their blueprints accurately captured their intentions.

In the second class, students recreated their blueprints, either using Minecraft or Lego blocks. They brought in their own computers if they were using Minecraft, and at one point we noticed that there were seven different kinds of computers in use. If they had Lego at home, the students brought that in as well, and extra Lego and building boards were provided for all other students.

The students had to use their blueprints and their models to calculate the area and perimeter of their designs, with some students also calculating the total volume, and explain their solutions. This task therefore assessed the students’ ability to create and read a blueprint, calculate perimeter and area, and communicate their understanding of mathematics. It also required them to demonstrate their visualization skills to transfer their 2-D plans to a 3-D structure, an area of mathematics that often falls second to calculations.

During the lesson, students were observed problem-solving using mathematics and applying mathematics skills they had previously learned. The students had to continue to develop their numeracy skills while at the same time starting some pre-algebraic reasoning, as they tried to get the scale and dimensions of their blueprint to match their constructed diagrams.

The context of the problem was realistic, so that students should have seen that mathematics is something that one can use in one’s life. The context helped students draw connections that they may have otherwise not understood; for example, the relationship between multiplying two numbers and the area of a rectangle. We hope this will keep students, and their teachers, inspired about mathematics!

Probably the best sign that this is a project worth doing actually came from the fifth grade students. When they heard that this year’s fourth grade class was breaking out the Lego, they said, “Oh, I remember doing that project. That was fun!” The fifth graders did a project that involved practicing skills, learning mathematics in context, and they not only remember doing it, they found it fun. That sounds like a success to me.

June 21, 2013 / 0 Comments

I will miss you

Yesterday was my last day working with students as a classroom teacher, at least for the foreseeable future. Next Tuesday is my last day of work in my current school, and on Wednesday I will be flying to New York to start a new job.

I will miss hearing piano music drifting into my office as I work.
I will miss people asking me questions about all sorts of things.
I will miss the sound of students working and playing.
I will miss wandering around our quirky but lovable buildings.
I will miss standing on the street corner to make sure kids are safe.
I will miss our kids.
I will miss calling a group of people who are in no way “mine”, “my” kids.
I will miss discussing the big (and small) ideas with students.
I will miss random treats in the staff room.
I will miss grateful letters from parents and students.
I will miss grad pranks and graduation dinners.
I will miss taking kids on field trips to see exciting things.
I will miss heart-felt discussions about life with a group of people that has hardly lived any of their own life yet.
I will miss seeing an aha moment when someone gets it.
I will miss watching a group of kids grow and mature.
I will miss the bustle of a class working on a project that has meaning to them.
I will miss being surprised by what students can do.
I will miss my friends.