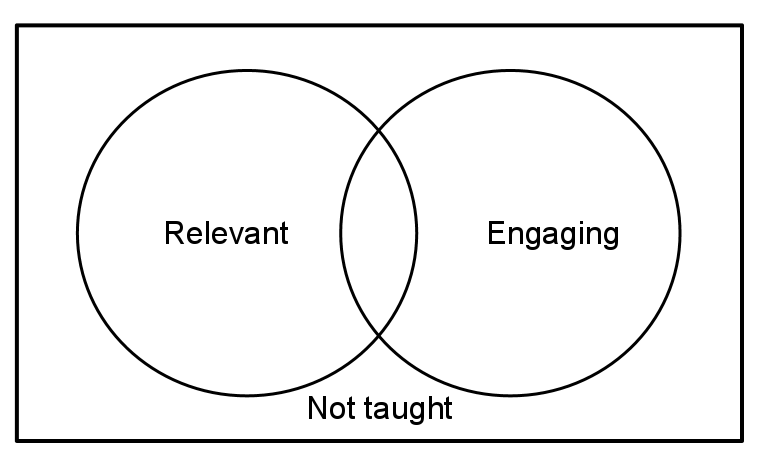
What you teach should either have relevance in your students lives, either now or in their future, or it should be engaging. If neither of those is true, don’t teach it.
Education ∪ Math ∪ Technology

What you teach should either have relevance in your students lives, either now or in their future, or it should be engaging. If neither of those is true, don’t teach it.
Nick says:
I think this is an over simplification of a very complicated issue. What does relevant mean? Does it mean I can relate to it; that it has some obvious relevance to my everyday life? If that’s the case a lot of very important math that does not fall in the union of engaging and relevance.
For example: limits. Limits have no obvious relevance to my life, and they’re definitely not engaging, but they’re a necessary prerequisite to calculus (which is relevant and engaging). The same goes for factoring polynomials, trig identities (though I think those are engaging), and logarithms. Not relevant or engaging in the short term, but hugely important in the long term.
Perhaps a third set belongs in your Venn Diagram… maybe significance or importance?
June 28, 2011 — 9:46 am
David Wees says:
Limits do have a relevance for your future life, especially if you are planning on learning calculus. When teaching limits, you can make them much more engaging by telling the stories that summarize the history of trying to understand limits. For example, I talk about the problems the Pythagoreans had with limits, Xeno’s paradox, and other related stories. This give students some historical context of limits, and that combined with understanding why they will be important to them later means that they can be both engaging and relevant.
Now the corollary is that if a student is not going to be taking calculus, they shouldn’t learn limits, because as you suggest, they are otherwise a pretty irrelevant topic for students.
June 28, 2011 — 11:57 am
Nick says:
I agree that limits have long term value, as stated in my comment, “…they’re a necessary prerequisite to calculus (which is relevant and engaging).”
What I’m challenging is the vagueness of the word “relevance.” Do you mean relevant to my personal life? My professional life? My mathematical future? From your reply, I judge you mean that last.
I do rather like your suggestion of using Zeno’s Paradoxes to make limits lectures engaging.
June 30, 2011 — 10:38 pm
David Wees says:
I’m not really convinced that calculus is either relevant or engaging for most high school students. I loved learning calculus myself, so of course I found it highly engaging. I think it’s a stretch to take what passes for calculus in most schools and call it relevant. In fact, in many ways the fact that we put calculus at the top of the pyramid of mathematical knowledge for grade 12 students leads to a lot of fairly uninteresting mathematics being taught.
While calculus is a terrific way to describe the universe, it is very rarely taught as a tool for understanding, and more often taught as a series of calculus which "you’ll find useful later." How many calculus teachers talk about the rivalry between Newton and Liebniz. How often do students get to attack actual problems with calculus rather than canned pseudo-problems?
July 1, 2011 — 10:39 am
Nick says:
It’s the “you’ll find useful later.” sentiment that I’m very much concerned about. You seem to be saying that it’s ok to say that when you’re teaching limits (to calculus bound students), but not ok in a calculus class.
And while I do find math history interesting, I think calculus, by sheer elegance and beauty, is engaging on its own. This may just be because I’m a huge nerd, but the Fundamental Theorem of Calculus, Taylor series and even the notation all gave me chills when I first learned them. Actual problems, no matter how relevant to my life, are usually just a snore fest for me.
I realize that I’m most likely in the minority here (though there are other unabashed mathaphiles out there), but like G.H. Hardy, “I am interested in mathematics only as a creative art.” And this brings me back to my original point: I think many educators are looking for the one size fits all solution for how to get students excited. But what will engage most students, will bore some. What is relevant to me, might not be relevant to you. So what then are the definitions of “relevant” and “engaging?”
I don’t suggest that I have the solution (or even that there is one), but only that I don’t think it’s as simple as this Venn Diagram indicates.
July 1, 2011 — 2:21 pm
David Wees says:
I freely admit that this Venn diagram is gimicky and an over-simplification of the issue. Obviously we need to ask questions like, relevant to whom, and how do we define engaging? However, the point is to get people thinking about what they teach and if they can’t obviously fit it into either of these categories, if they can’t justify their curriculum, then it needs to be rethought.
July 2, 2011 — 12:29 am