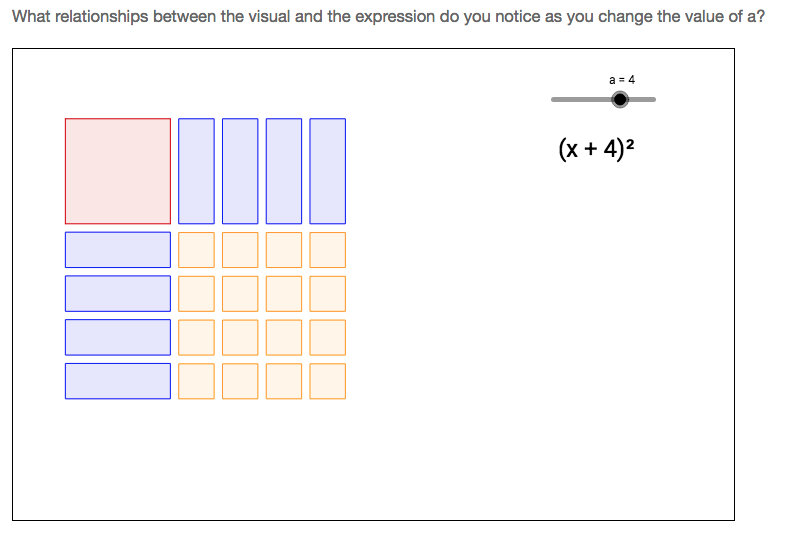
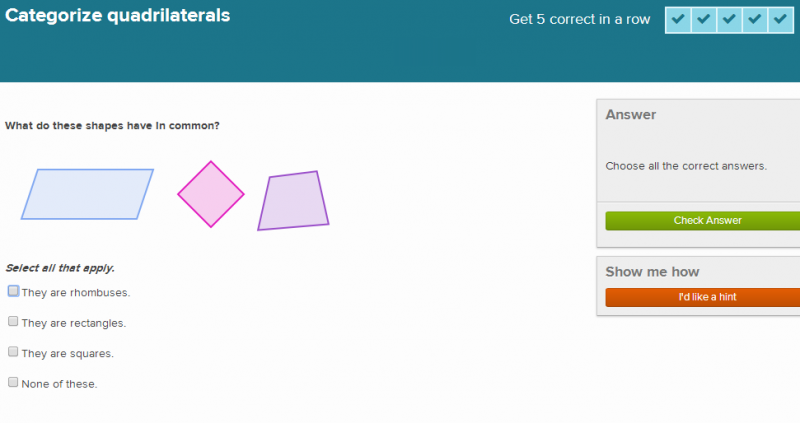
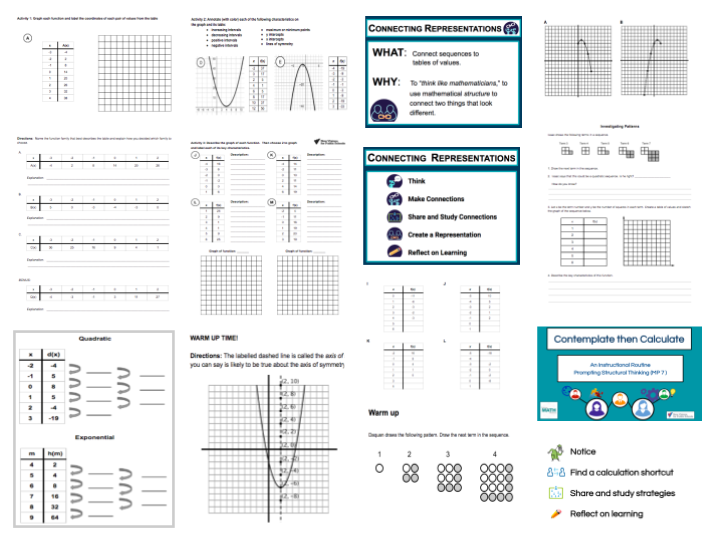
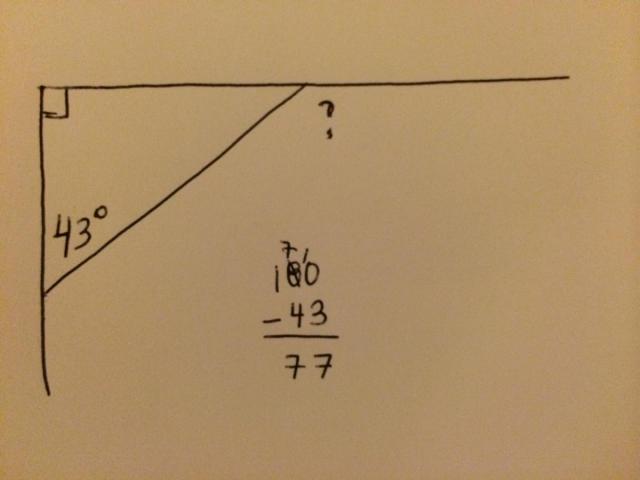When someone is learning a new practice, it is common to isolate that practice from other elements of the greater body of work they are also learning. For some areas of learning, this is easier to do than others. For example when learning how to play the piano, one can reasonably easily practice scales and parts of songs and then integrate those parts into the whole.
In learning teaching however, since every practice is connected to other teaching practices, it can be extremely challenging and potentially unhelpful to isolate individual teaching practices. For example, you cannot really get better at the 5 Practices (summary, book) without considering how those 5 Practices interact with each other. If you anticipate student ideas for an upcoming lesson, you will only get feedback on that anticipation if you also monitor what students do.
One strategy to reduce the complexity of learning to teach is to approximate teaching practice in various ways. Instead of teaching a whole class of students, one can teach at one table. Instead of teaching five classes of students a day, one can teach one class. Instead of teaching on one’s own, one can co-teach with a mentor teacher.
Another approximation of teaching we have found helpful in our work is the use of an instructional rehearsal, which is where one teacher (or perhaps a pair of co-teachers) leads the group in a teaching experience with everyone else playing the role of students. At either strategic instances or on request of the teacher(s) leading the experience, the action stops and everyone considers teaching practice either together or in small groups.

Rehearsals: A common practice in many disciplines
It is helpful for one person to act as the facilitator or coach for this experience, and for the rest of the participants to switch between playing the role of students while the teaching experience is in action, and to discuss the teaching as teachers when it is not. If each rehearsal has a different focus, then one can learn different elements of teaching over time, while still maintaining the complexity of teaching. The goal is for the core practices of teaching to become integrated rather than overly isolated. The Teacher Education by Design website has more details and resources for instructional rehearsals here.
A further design element of instructional rehearsals is that the activity to be rehearsed should be fairly clear for participants. We use instructional routines as the frame for our rehearsals because they constraint the scope of potential decisions to be made and subsequently discussed but are still complex enough examples of teaching to allow for different foci or teaching practices to be discussed in different rehearsals. We typically model an instructional routine a few times for teachers, unpack it collaboratively, then teachers plan around a task for the instructional routine, and then rehearse the instructional routine one or two times as a whole group.
Rehearsals can be places to discuss planning processes and protocols that might be necessary pre-steps to improve the enactment of a performative teaching practice. For example, while considering how to annotate a student strategy during a rehearsal, participants will likely realize that practicing different annotation strategies in advance of a lesson would be helpful and that in order to do this, one should first anticipate the student strategies that are likely to emerge for a particular.
We have found rehearsals to be helpful for teachers at all stages of their career, since all teachers have room to grow and to learn. The foci of the rehearsals for pre-service, early career, mid-career, and late career teachers may be different but the overall process is the same.
One other key idea of rehearsals: the goal is rarely to give the teacher leading the rehearsal feedback although that often happens but to collaborate together to consider teaching. The goal is to collectively improve teaching practice not individual teachers.
Rehearsal is not a replacement for working with a mentor teacher over time to learn ways to communicate with parents and other critical aspects of the role of a teacher. Some elements of teaching practice are hard or potentially impossible to rehearse. However the performance aspect of teaching is where most teachers will spend at least half of their time, and rehearsals are a good strategy for developing performative teaching practice.
References:
Kazemi, E., Franke, M., & Lampert, M. (2009). Developing pedagogies in teacher education to support novice teachers’ ability to enact ambitious instruction. In Crossing divides: Proceedings of the 32nd annual conference of the Mathematics Education Research Group of Australasia (Vol. 1, pp. 12-30).
Knowing Teaching from the Inside Out: Implications of Inquiry in Practice for Teacher Education. (1999). In G. A. Griffin (Ed.), The education of teachers (pp. 167-184). Chicago, IL: NSSE.
Lampert, M. (1990). When the Problem Is Not the Question and the Solution Is Not the Answer: Mathematical Knowing and Teaching. American Educational Research Journal, 27(1), 29-63. doi:10.3102/00028312027001029
Lampert, M. (2009). Learning Teaching in, from, and for Practice: What Do We Mean? Journal of Teacher Education, 61(1-2), 21-34. doi:10.1177/0022487109347321
Lampert, M., Franke, M. L., Kazemi, E., Ghousseini, H., Turrou, A. C., Beasley, H., . . . Crowe, K. (2013). Keeping It Complex: Using Rehearsals to Support Novice Teacher Learning of Ambitious Teaching. Journal of Teacher Education, 64(3), 226-243. doi:10.1177/0022487112473837
Lampert, M., & Graziani, F. (2009). Instructional Activities as a Tool for Teachers’ and Teacher Educators’ Learning. The Elementary School Journal, 109(5), 491-509. doi:10.1086/596998
Mcdonald, M., Kazemi, E., & Kavanagh, S. S. (2013). Core Practices and Pedagogies of Teacher Education: A Call for a Common Language and Collective Activity. Journal of Teacher Education, 64(5), 378-386. doi:10.1177/0022487113493807