(Image credit: toomuchdew on Flickr)
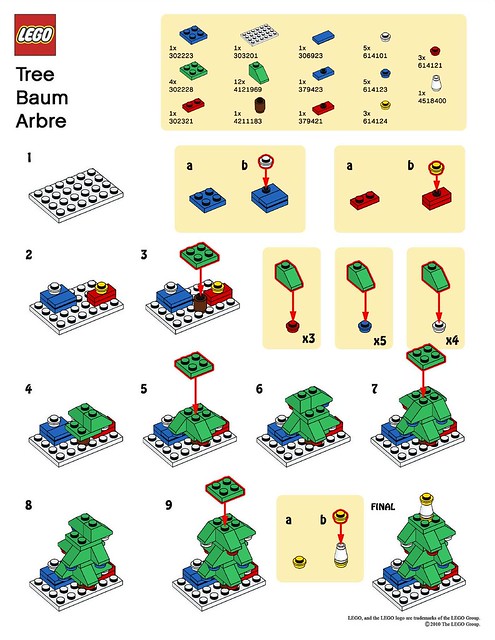
When I was a kid, I had a lot of Lego, most of which was given to me as birthday gifts, and came in nice neat boxes with instructions on how to build whatever was on the picture on the outside of the box. I would often follow the instructions carefully once, to make sure I could create the picture, and then that package of Lego joined the big bucket of Lego, and I never assembled that particular design again. I did spend a lot of time creating my own models, and learning different ways of putting the pieces together myself. I remember better the things I created than the things I assembled. 25 years later, I still remember when I used all of my Lego to create a sprawling metropolis on my floor.
My son and I play Lego together, and just like when I was a child, he wants to follow the directions. We’ve built a couple of things together that way, but we’ve also spent hours upon hours making our own designs. Recently, we’ve been constructing sling-shots with our Lego along with some rubber bands, and then using these to fire smaller pieces of Lego at targets. My son calls this "playing Angry birds." In this case, we’ve had a design (the Angry Birds slingshot) and we’ve reverse engineered a way to create this slingshot with Lego.
We need to be careful that we give students time for creation, which I see as a much different process than assembly. Creation helps kids develop entrepreneurial skills, use their imagination, and allows them to be inventors. Assembly allows them to learn how to follow instructions, and work toward a well established goal. There is also a middle ground between the two, which I call reverse engineering where you give students the final outcome, and they have to work to figure out how it was built.
When you assign a project and give students a highly structured way of completing the project, you are having them assemble their project, rather than create it. They may not be assembling pieces of Lego, but it is a form of assembly nonetheless. These kinds of projects can be valuable for your students, and can help focus students on the final product of the project but the more information you give students on what their project should look like, or how they should go about doing it, the less freedom they have to actually be creative.
There is research which shows that when kids are shown how to do something (as what happens when you give them a set of instructions to follow), they are less likely to "engage in spontaneous exploration and discovery" (Thanks to @jybuell and @andymikula for sharing those two links). We must then be very careful about our purpose in giving kids a set of instructions to follow, given that we may be shutting down some of their creative capacity in this area.
There are types of tasks for which instructions are pretty important. You can’t write a high quality academic paper without reading examples of other academic papers, and without some pretty careful instructions on formating. The language of academic papers is highly specialized and often cryptic.
However, I don’t want my son writing academic papers just yet. He’s young. I want him to explore the world, and see what is possible, and use his imagination as much as he can at this young age (thanks @allanalach for this link). He has plenty of time to learn about the dydactic academic world later. For now, I want him to play.
A subject like science, for example, can very much be taught either as an act of creation, or as an act of assembly. Give students all of the instructions on how to complete a lab, and they are assembling a lab. Give them a goal (figure out why this phenomena works) and they are reverse engineering. Give students time to play (safely) with the tools in a laboratory and come up with their own experiments, and they are creating.
Scientific inquiry is about asking questions and exploring the answers to those questions through experimentation. If we want kids to think like scientists, we need to give them the ability and option to experiment. As this comic suggests, the core of science is that ideas are tested by experiment, and that everything else is just bookkeeping.
Let’s make sure that our need for bookkeeping doesn’t disrupt our kids need for exploration. Let’s make sure we give kids lots of time to be creators, and not have them just assemble stuff.