Month: March 2016 (page 1 of 1)

If you or your students are going to talk about mathematical ideas in your class, it is critical that everyone understands the idea being discussed otherwise they are less likely to either remember it or be able to participate in the discussion.

Every time you or your students make logical leaps when explaining mathematical ideas, your students must fill those logical leaps with what they understand about mathematics or invent their own logic to fill the gaps.

Consider this task:

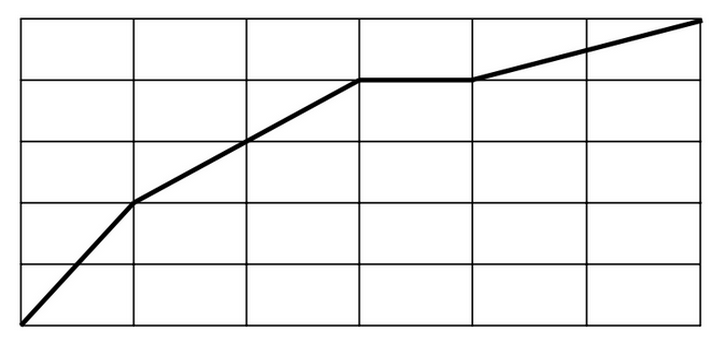
Which line segment is steepest? (source)

Make the task explicit

This task as currently written is actually ambiguous. There are a lot of vertical and horizontal line segments in this picture; are they meant to be included, or not? Are we only supposed to focus on the bold line segments?

We could change the prompt to something like “Which of the bold line segments is steepest?” This of course assumes that students understand what a line segment is and interpret bold to mean the same thing their teacher means. (It’s fine if students don’t completely understand what steepest means though so since the goal of a task like this is to come to a common definition of steepest.)

Use Gestures

Another approach is when asking students to solve the task, having it projected on a screen, and using one’s hand to trace and emphasize the line segments in the image, while asking the question.

Push for clarity

Now consider these (simulated) student strategies for solving this task and imagine students are describing their strategies out loud to share with the class.

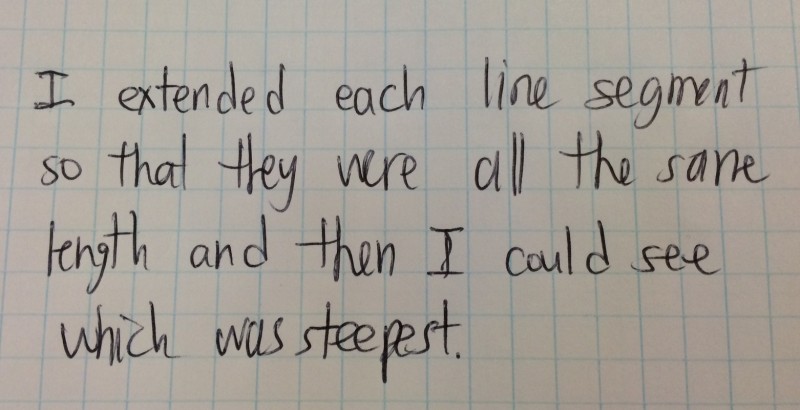
Student 1

Student 2

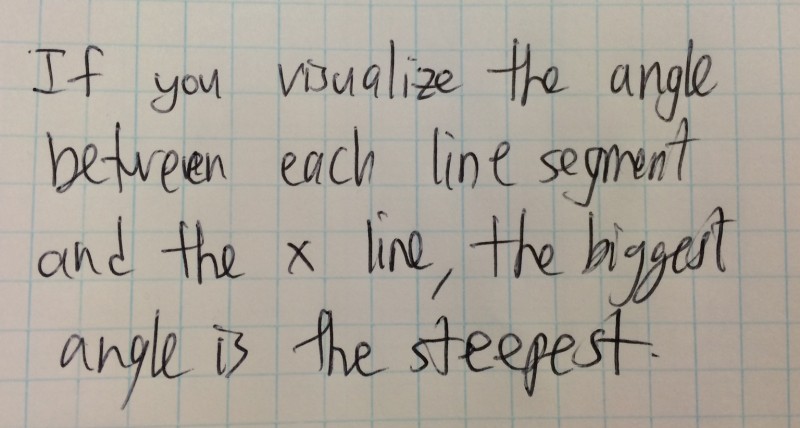
Student 3

If you have taught students how to interpret lines or line segments on a graph (or remember the mathematics associated with the task from when you learned it), you can probably figure out what these strategies mean. But there are gaps or missing steps in each explanation and since the explanations are out loud, there are ambiguities in each explanations as well.

With respect to the first strategy presented, what does it look like to extend all of these line segments so that they are the same length? How does the first student know that just because the line segments are now the same length, that one of them is steeper than another? And which line segment did they actually find to be steeper anyway?

In the second strategy, where are those little triangles drawn? Are they connected to the line segments in some way? And even if they are, one of the line segments is horizontal; how do I draw a triangle under that? Why does the largest rise over run correspond to the steepest line? Is that always true?

In the third strategy, where exactly are the angles between the line segments and the x-line? And what is an x-line? And why does the largest angle correspond to the steepest line segment?

Use questioning

One strategy is to ask clarifying questions about the strategy or to prompt students to ask clarifying questions of each other. In order to be able to ask critical questions “on the fly” it is extremely helpful to have anticipated the approaches students will use and at least some of the possible leaps in reasoning they may make, so that you can prepare questions in advance.

Use annotation

If you or students talk about mathematical ideas with no public written record of what was discussed, chances are high some students will either not be able to follow the argument being made or will quickly forget the argument. You can, and should, keep this record for students during discussions and use color and symbols to make the connections between mathematical ideas clear.

Here are some examples of annotations related to the student strategies above. Do these annotations make the ideas being discussed more clear? Is it more obvious why these strategies work?

Annotation for Strategy 1

Annotation for Strategy 2

Annotation for Strategy 3

Keep a public record

Here is a record of what participants noticed and what their meta-reflections were when I used this task with them.

What participants noticed

What participants reflected on

Having this public record means that if a student’s attention wanders, they can get back into the flow of the class. It also means the information you want students to take away with them remains up for as long as possible. Further, when you move to prompting students to consider why the mathematical strategy works, students’ cognitive load around what the actual strategy being considered is decreased while there is a public record that they can access.

Prompt students to consider each others’ ideas

Unfortunately, usually when people (our students included) listen to each other they listen for what they expect to hear rather than what was actually said.

While it is worth saying that you should actively listen to what students say rather than changing its meaning or filling in the gaps in logic yourself, you will also want to use talk moves (like revoicing, restating, asking a student to restate, asking questions, wait time, etc…) to push your students to actively listen to each other as well.

Use independent think time

When we run professional development sessions, we virtually always incorporate a section on doing the math as this increases the odds that our teachers are able to have meaningful discussions about how to teach the mathematics. If students have already thought about a problem themselves, they will find it easier to understand someone else’s approach more easily.

What are other ways we can make the mathematics in our students’ strategies explicit while simultaneously respecting the thinking that students put into these strategies?

In our project, we organized our work this past year around the use of instructional routines (née instructional activities) with teachers. Our curriculum work has been largely focused on instructional routines, our professional development activities have been focused on instructional routines, our school-based work in some cases has shifted to focus on supporting teams using instructional routines together. Our objective this last year has been to develop teachers in teaching ambitiously through the use of instructional routines that embody this kind of teaching.

Instructional [routines] are tasks enacted in classrooms that structure the relationship between the teacher and the students around content in ways that consistently maintain high expectations of student learning while adapting to the contingencies of particular instructional interactions.

Kazemi, E., Franke, M., & Lampert, M. (2009)

Instructional routines are a little bit like a standard play in sports. If everyone knows the play, then the game runs more smoothly. But just like when in sports plays have to be adjusted due to what’s going on in the game, instructional routines have to be adjusted on the fly due to the ideas that emerge from students in the classroom.

Here is an example of a task intended to be used in an instructional routine called Contemplate then Calculate.

Find the number of grey squares in the next term of this pattern.

The instructional routine Contemplate then Calculate has (roughly) these five steps:

1. Launch: The teacher launches the routine to let students know what, why, and how the class will be proceeding.
2. Noticings: The teacher flashes an images for kids and asks kids to describe what they noticed in the image, share this with a partner, and then records some noticings for the whole room to use.
3. Partner work: The teachers reshares the image with a problem task associated with it, then kids work with a partner to solve the problem given.
4. Share: Selected students share their strategy with the whole class while the teacher annotates the strategy and uses talk moves like restating and probing questions to ensure that everyone understands the ideas being presented.
5. Meta-reflection: Students write reflections based on choosing from prompts given to them by the teacher.

The high level goals of Contemplate then Calculate are to support students in surfacing and naming mathematical structure, more broadly in pausing to think about the mathematics present in a task before attempting a solution strategy, and even more broadly in learning from other students’ different strategies for solving the same problem. A critical aspect of this and other instructional routines is that they embody a routine in which one makes teaching decisions, rather than scripting out all of the work a teacher is to do with her students.

This year we noticed a number of benefits to using instructional routines that lead us to plan continuing using them next year as well.

1. Instructional routines allow teachers to communicate about classroom practice with each other using a common language and common understanding of what kinds of instructional strategies are being implemented.

Usually conversations about classroom practice are extremely difficult because each teacher’s context is so different and because teachers visit each other so infrequently. My experience suggests that these conversations often devolve from talking about specific decisions that were made and the rationales behind those decisions and into discussions about mathematical topics and what order they should be taught. With a common instructional routine, teachers’ conversations can shift to a more granular level of discussion since so much more of the context can be assumed.

2. Instructional routines can support teachers and students in having access to high cognitive demand tasks by reducing the cognitive demand needed to attend to “what am I doing next”. Since the activity is routine and well-defined, the steps to doing the activity can fade into the background over time for both teachers and students. This allows more of the cognitive load for teachers and students to be potentially focused on making sense of each others’ reasoning and the mathematics of the task at hand.

Shifting cognitive demand for teachers and students

Teachers already have many routines they use in their classrooms but those routines may or may not be used by other teachers (see point #1) or they may have too many different routines that they enact for each type of mathematical task they use. In order for the cognitive benefits of an instructional routine to occur, the instructional routines must in fact become routine.

3. Instructional routines have allowed our curriculum team to rapidly develop mathematical tasks to fit into these instructional routines because we don’t need to communicate the routine separately for every task. The routine stays the same (but see point #4) over time while different tasks are enacted within the routine.

4. Since an instructional routine keeps much of the classroom interaction the same, it becomes possible for individual teachers or groups of teachers to iterate on their practice more rapidly. If every day a teacher has to re-invent her practice, then it becomes more difficult to figure out what teaching strategies work in her context, when those teaching strategies work, and why she might choose a different teaching strategy.

I remember my first year teaching. I was unprepared. I didn’t know how to structure lessons. Each day I was floundering. I kept experimenting and trying different activities, different ways of communicating with students, etc… I would have benefited from more support in planning lessons.Note that this benefit supports newer and experienced teachers differently. A new teacher needs a starting place to iterate on their practice from. An experienced teacher who wants to refine her practice needs a tool with which to do so.

5. In professional development settings, we and teachers in our project can model teaching strategies more easily (this is really a combination of point #1 and #4). Since the routine is well-established, when someone does something different within the routine when modeling it with a group of teachers, it becomes easier to focus on the something different.

For example, we used the routine Contemplate then Calculate to model instructional moves intended to facilitate student discussions. We then, as a group, unpacked just that aspect of the routine. This was enabled because instead of everyone participating having to keep all of the teaching occurring in one’s head at one time, the routine aspects of the teaching could be ignored to focus on the non-routine aspects for that day.

6. The instructional routines all include built in opportunities for formative assessment and responsive teaching. We struggled to find ways to staple formative assessment practices on top of existing teaching and mostly failed. Instead the different aspects of formative assessment (as described by Dylan Wiliam) are embedded within an instructional routines, which as it turns out, makes them easier to learn how to use.

There are other more mathematical benefits of these instructional routines, but those depend largely on the specific routine being used.

We intend to continue supporting the two instructional routines we used this year (Contemplate then Calculate and Connecting Representations) and to add one or two more routines to support different mathematical goals teachers may have, because we have seen each of the benefits of these routines listed above play out in various ways across our project.