Month: December 2009 (page 1 of 1)

So a while back I posted a link to an survey I conducted.  I didn’t have an enormous amount of respondents, but I’ll share the results with you.

First it should be noted that there is some selection bias.  Actually probably LOTS of selection bias, given that this survey was conducted entirely online and that people who read this blog, or found the link to the survey through Twitter are probably pretty IT savvy.  That being said, you might still end up being surprised with the results.

There were two questions on the survey.  

1.  How many sheets of paper (approximately) do you use in a day?
2.  How many teachers do you have in your school?

The lowest answer to the first question was 1 single sheet of paper a day (good for you!) and the highest was 75.  The lowest answer to question number 2 was 6 teachers and the highest was 170.  The 11 respondents used a total of 326 sheets of paper a day, or just over 26 sheets each.  Probably this is pretty good, I would expect that a typical teacher probably uses more.

According to these results, a typical school in which these respondents work uses about 2000 sheets of paper a day, or about 360,000 sheets in a school year.  Of course there are thousands of schools in Canada and the US (where most of the teachers who responded probably live), 96,000 or so in the US (or 120,000 depending on who you believe) and this means that more than 34 billion sheets of paper are used each year just in the United States.  Assuming that each sheet costs a mere 5 cents (photocopy paper at Office Depot apparently costs 38 cents) a sheet, then to provide paper to every child in the US each year for school costs about 2 billion dollars a year.

For comparison, providing each child in the US with a $100 laptop (recently available on the market) would cost about 8 billion dollars assuming even the little kindergarten children get one.  In other words, we could pay for a laptop per child in the US in 4 years by stopping using paper in schools.  Oh and that laptop can also replace the paper…

Of course if the people surveyed are far from standard, then maybe schools actually use twice as much (or even four times as much) paper, in which case the amount of time it would take for the savings from not using paper to turn into laptops would be reduced to half (or even a quarter) of the time estimated.  In other words, we could potentially turn billions of pieces of paper into every child in the US having a laptop.

Now I’m using US numbers here for this calculation (because the supporting figures are easier to find) but it shouldn’t take you long to realize that this is probably true of any industrialized nation with similar expenditures on paper.  Perhaps we can use some financial arguments to persuade our legislators to put some good tools into the hands of our students?

I’m working on a simple project to explain to students the importance of learning integration (which is an important technique in Calculus).  The basic idea is this, take a model of something from the real world, like a car for example, and find the area represented by the model.  You can talk about the importance in figuring out how much the metal will cost to make the car, or how much paint is required to cover the car, etc…

Here’s how we are going to do it in my class.

First we use our friend Google Image search and find a model.  I found some great models from http://carblueprints.info and settled on a 1931 Ford Window Coupe as an example.  It’s not clear to me what license these images are under, but given that I am using them exclusively for educational purposes and that there is no commercial value in what I am doing, I’m probably okay to use them here.

Once I had an image, I cropped it down somewhat and resized it.  This was to make it more convenient to embed in my favourite graphing program, Geogebra.  You could very easily use any graphing program here, or even print out the image and do the rest of this project on paper.

The next step was to open up Geogebra and insert the image.  Lots of tutorials on how to do this and push the image into the background, and of course the steps will vary depending on which program you use.  Once I had the image in Geogebra, I added points around the edge of the image at the critical parts of the model.  I basically want to split the edges of the model into the different functions.

Once you have the coordinate point, which are the difficult things to find really, you can now use all sorts of techniques to find the functions.  For example, which a less advanced class, they could approximate the shape given using straight lines.  For a more advanced class, they could use lines, circles, polynomials, whatever to find the functions which represent the shape of the curves.  

One nice option here is to either use the regression tool on a graphing calculator, or available in Excel to find the functions as appropriate.

Once you have the many functions which represent the shape, the students will have to find the x bounds of the functions (which should be easy if they have recorded the coordinate points) and then integrate each function over the appropriate bounds.  One thing that could get students stuck at this stage is remembering that if they actually want to find the area ABOVE the curve, they will need to either flip their function over the x-axis, or more easily, find the absolute value of their negative answer for the integration of that function.

This activity allows for all sorts of differentiation (in terms of pedagogy, not calculus!) as well.  Students who might struggle can be encouraged to choose easier models to begin with.  You can also point out that some areas of the shape might be better done using actual area formula instead of integration or potentially this same type of activity could be used at a much lower level of mathematics using just area formula.  These models represent excellent examples of composite areas, and their realism will help students recognize the relevance of what they are learning.

I haven’t actually gone through the entire process of the integration itself.  I’m going to be using this activity with my students today and I don’t want to give it all away yet!  Wait for me to post some examples of their work when they finish.

 I’m writing this post, inspired by Tim Gower’s Massively collaborative mathematics project.  The basic premise Gowers takes in his essay is that the power of many minds, working collaboratively, is much greater than a single mind, even in a highly technical field such as mathematics.  Gowers proposed working on a particular problem in mathematics, and according to third party reports I read, the team of mathematicians that participated solved the problem in just six weeks.

So I asked myself, in what way could we replicate this process in education?  It seems to me that much of educational theory contains large and intractable problems which have many different possible theories.  It should be a perfect place to test the collective power of educators to solve problems.

I don’t know if this is exactly the right problem to start with or not, but I had a complete idea about it, so I’m starting with it.  If someone thinks some other area is more important, please produce a comment with your own complete rebuttal and we can come to some agreement about which way to proceed.

The basic rules we should follow I think should closely follow Gower’s own rules taken verbatim from his website:

1. The aim will be to produce a proof in a top-down manner. Thus, at least to start with, comments should be short and not too technical: they would be more like feasibility studies of various ideas.

2. Comments should be as easy to understand as is humanly possible. For a truly collaborative project it is not enough to have a good idea: you have to express it in such a way that others can build on it.

3. When you do research, you are more likely to succeed if you try out lots of stupid ideas. Similarly, stupid comments are welcome here. (In the sense in which I am using “stupid”, it means something completely different from “unintelligent”. It just means not fully thought through.)

4. If you can see why somebody else’s comment is stupid, point it out in a polite way. And if someone points out that your comment is stupid, do not take offence: better to have had five stupid ideas than no ideas at all. And if somebody wrongly points out that your idea is stupid, it is even more important not to take offence: just explain gently why their dismissal of your idea is itself stupid.

5. Don’t actually use the word “stupid”, except perhaps of yourself.

6. The ideal outcome would be a solution of the problem with no single individual having to think all that hard. The hard thought would be done by a sort of super-mathematician whose brain is distributed amongst bits of the brains of lots of interlinked people. So try to resist the temptation to go away and think about something and come back with carefully polished thoughts: just give quick reactions to what you read and hope that the conversation will develop in good directions.

7. If you are convinced that you could answer a question, but it would just need a couple of weeks to go away and try a few things out, then still resist the temptation to do that. Instead, explain briefly, but as precisely as you can, why you think it is feasible to answer the question and see if the collective approach gets to the answer more quickly. (The hope is that every big idea can be broken down into a sequence of small ideas. The job of any individual collaborator is to have these small ideas until the big idea becomes obvious — and therefore just a small addition to what has gone before.) Only go off on your own if there is a general consensus that that is what you should do.

8. Similarly, suppose that somebody has an imprecise idea and you think that you can write out a fully precise version. This could be extremely valuable to the project, but don’t rush ahead and do it. First, announce in a comment what you think you can do. If the responses to your comment suggest that others would welcome a fully detailed proof of some substatement, then write a further comment with a fully motivated explanation of what it is you can prove, and give a link to a pdf file that contains the proof.

9. Actual technical work, as described in 8, will mainly be of use if it can be treated as a module. That is, one would ideally like the result to be a short statement that others can use without understanding its proof.

10. Keep the discussion focused. For instance, if the project concerns a particular approach to a particular problem (as it will do at first), and it causes you to think of a completely different approach to that problem, or of a possible way of solving a different problem, then by all means mention this, but don’t disappear down a different track.

11. However, if the different track seems to be particularly fruitful, then it would perhaps be OK to suggest it, and if there is widespread agreement that it would in fact be a good idea to abandon the original project (possibly temporarily) and pursue a new one — a kind of decision that individual mathematicians make all the time — then that is permissible.

12. Suppose the experiment actually results in something publishable. Even if only a very small number of people contribute the lion’s share of the ideas, the paper will still be submitted under a collective pseudonym with a link to the entire online discussion.

In order for this to work for an educational research paper, some of the rules need to be slightly modified.  For example, in rule number 1, we need to change the word proof to something else more appropriate.  Rule #12 in my opinion should be that everyone who participates has their name attached to the study, however this may end up offending some journals given that if 500 people participate, the list of authors alone will take a couple of pages!  However most of these rules deal with the social interactions which occur for such a project, and in this sense they are applicable.  We’ll follow these rules keeping in mind that some of them will need to be slightly rewritten, and of course you are welcome to consider other ways of formulating the rules and we can come to some agreement on the reworded rules.  

My question is, "Does assigning and collecting homework in mathematics lead to greater retention of material among students?"  It’s a question which has been answered both ways many times each and which could considered to be unsolved given the controversy surrounding it.  So I’d like to try and answer it definitively.

Here is what I would outline as the steps we would need to undertake in order to answer this question.

1.  We need to recruit other people to help us with this project.  So far it has one mathematics teacher involved (myself) and will make a very weak study!

2.  Each teacher who is involved should be working on the same unit, using the same lesson plans, and assigning the same homework for the same lessons.  We want to ideally eliminate other sources of differences in student’s performance on the final test.  To this end, we need to decide on a common unit, create a pretest and a post-test for this unit, and then generate the lesson plans necessary with the accompanying homework.  Half of us will teach the same unit with the homework, the other half without.

3.  Once a teacher is ready to report results, they should do so with their name included in the results, but no other identifying information about the students.  We may even need parents to sign waivers in certain districts, although it is my understanding that there are many types of educational research that can be done without parents permission, this may be one of them.

4.  Everyone can do the units at different times, so long as they follow the same structure.  To make this more feasible, the unit we choose should be one that is taught as widely as possible and which can be independent from other units in a mathematics course.  Although as I write this I am concerned that we may be actually answering a narrower question, "Does assigning and collecting homework in this single unit of mathematics lead to greater retention of material among students?" 

A fringe benefit of this exercise is that everyone who participates will have all of their lessons and homework prepared for an entire unit, which may make the work of preparing results and submitting them feel a bit less onerous!

Please let me know if you want to participate in this study, remembering that everyone who participates will benefit from this study.  Also let me know if you think a different question (or procedure) is better and give me reasons why you think this is true.  My interest in this project is mostly in the WAY in which we are going to proceed rather than what we actually accomplish.

The problem:

Passwords are ubiquitous today.  Everyone who spends any time online has many different passwords they need to remember.  Unfortunately the more passwords you have to remember, the more likely it is that you will forget some of them.  Some people get over this complication by using a bunch of different but simple to remember passwords, other people use a complicated password for every website.

Both of these are the wrong way to go and can expose yourself to risk online.  The simple passwords can each be individually hacked very quickly by even a slow desktop computer.  For the complicated password, it is harder to hack, but much more likely to cause more problems if you lose it some other method.

Bad:

First, your password should not be something that could be found in a dictionary (any dictionary).  The total number of words in the English language, including scientific terms, is about one million.  This means that a typical desktop computer would take about a second to run through all of the passwords, and your password could be cracked (on a website which doesn’t prevent brute force attacks) in under an hour, assuming standard load rates on the pages as the script attempts each possible password for the website.

Better:

Suppose you only use lower case letters for your password, and randomly choose the letters.  You might use a standard password of 5 letters long, in which case you’d have 26⁵ = 11881376  possible combinations, or just over a million combinations.  Obviously one way to make this password more secure is to increase the length of the password which multiplies the number of possible passwords (and the length of time to find them) by 26 for each extra character.

Another way to increase your password security is to increase the number of characters in your password.  Just by making your password case-sensitive and adding both UPPER and lowercase letters you double the number possible of combinations the computer has to check.  Throw in some numbers and some punctuation and you end up with a super secure password like: 94Gh-  Total number of possible password combinations in this case?  About 70⁵ = 1680700000 combinations or about 1.7 billion different combinations.  Total time for a computer to break?  Probably at least several weeks or even months, even with a fast computer (assuming that the hacker has to access the internet through an HTTP connection each time to check the password).

The advantage to this password is that it is relatively secure and not too hard to remember.  You could increase it in length to increase the complexity, each added character multiples the length of time it takes to hack your password by a factor of about 70.  So the more characters you can memorize the better.

The problem with this password is that if you use it on every website, if you lose it once (for example by a phishing scam where someone sends you an email and you click a link in the email and "log in" to a fake site) then you’ve just lost your password for every site you use!  Not good.

Solution:

The solution is, for every password you create, have a portion of it which is related to the domain (or function of the domain even) so that you can modify your standard complex password for every website, and still be able to remember all of these passwords.  So for example the password above might become 94Gh-f for Facebook, 94Gh-y for Yahoo, 94Gh-h for Hotmail, etc…  The best part here is, you can use a more complex algorithm which you can easily remember (like the second letter in the alphabet after the first letter in the domain), and create slightly different passwords which are extremely difficult for a hacker to figure out.

Update: As well, you should change your password somewhat regularly.  A teacher at my school commented that he changes his password everywhere once a year (you may wish to change it more often than this but being able to remember your password without writing it down is important so not too often).  How he does this is that he removes a character from the beginning of the password and adds a new character to the end of the password.  This way he keeps the same core password, but changes the password enough that it is difficult to crack, assuming it has been compromised on one of the sites you use it on.

Summary:

Avoid simple passwords as they can be cracked using a "brute force" attack.  More complex passwords can be created using more combinations of characters, upper and lower case, punctuation, numbers, etc… Memorize a difficult password and use it everywhere, modifying it slightly based on the url where it is being used.  For example use 94Gh-f for Facebook and 94Gh-y for Yahoo.

Update:

I strongly recommend also reading http://www.baekdal.com/articles/usability/password-security-usability/ which does a very thorough job of looking at the risks for passwords and has a different approach. Also, read this more recent article I wrote that talks about how to build easy to remember, but very secure passwords.

David Wees
University of British Columbia

(Download a copy of it here)

Abstract

The objective of this paper is to show that the costs of using educational technology are more than compensated by the savings schools can receive, provided the technology is introduced and used in the correct way.  So many schools are looking to trim money from their budgets, and this paper aims to show some of the ways this can be done.  The essential thesis is that productivity improvements will be where the majority of the savings will come from, with the remainder coming from conversion of the "old way" of doing things into the new cheaper technological method.

Introduction

Schools today, as always, are looking for ways to reduce costs. The current economic crisis and the recession of 2008 have hurt schools severely (Ring, 2008 & Tang, 2008) resulting in further cuts. This recession has impacted both public and private schools. Public schools rely on funds from taxes, which are always reduced in a recession since there is less income to tax. Private schools generally see enrollment drop during a recession as parents of their students lose their jobs due to the economic slow-down.

There are a few areas that schools can save money. Some schools cut services offered by the school, including dropping whole departments if necessary. Art and Music programs are disappearing all over North America as a result of the economic crisis and the No Child Left Behind law (Hetland & Winner, 2007).. Extra support services are also being removed from schools as school districts use "centralization" and "school sharing" as ways of reducing the hours school support staff are available at schools.

Technology has for many years been a focus of many schools. My current employment depends a lot on my talent with technology, in fact it is probably one of the primary reasons I was hired. This focus has probably developed as schools become more aware of the importance of what happens in a classroom reflecting what is happening in the real world. Without relevance to the real world, schools lose their value and certainly technology use is a huge focus of today’s world.

One of the ways that school can save money is by re-evaluating their current use of technology. Much of the money which is spent on educational technology is spent unwisely, with little thought given to the training technology requires or how the technology will actually be used.

A consistent trend in schools is that the way teachers teach changes as the schools adopt more current technology. Although schools are seen as resistant to change, over the years the opposite has been seen (Cuban, 1986). Schools have seen the introduction of radio, chalk boards, television, overhead projectors, internet, LCD projectors, interactive white boards, and most recently, mobile devices such as cell phones. Each of these devices comes with a cost which the school has to bear but has been shown to improve the education of the students within the school (Schacter, 1999).

There are many costs associated with this technology, some of which are purchase costs and can often be included as capital assets and others which are yearly costs and must be budgeted for each year. These costs can generally be categorized as either a hardware-related cost, or a training cost.

As computer hardware gets older, the cost to maintain the hardware increases. "A recent Gartner Group survey indicates IT budgets of companies worldwide amount to slightly more than 3.5% of revenue" (David, et al. 2002). Given that school board budgets, and even some school budgets, are usually in the millions of dollars, this cost is not insignificant. David, Schuff, and St. Louis (2002) describe how centralization and standardization can shave as much as 27% off of this maintenance cost.

In order to be able to do the standardization and centralization required to cut costs on IT, schools should have an expert on staff. Since the use of IT in education requires special considerations, this person should be both an expert in technology and an educator. The role of this person can also be to train staff in how to use this technology appropriately, which will save costs on hiring expensive educational consultants. As this person will be an integral part of the staff, they may be able to offset the “expert” phenomena, wherein teachers typically defer to the experts rather than learning the skills being taught themselves.

Another important observation to make is that cloud computing, in which information is stored on offsite servers and accessed through a web browser, can also greatly reduce costs for schools. Instead of paying for the cost of an onsite server and for maintenance of this server, one can run an entire school network offsite using cloud applications. One could use free cloud applications and replace almost all desktop software, saving an enormous of money in expensive licensing costs.

The cost of training staff how to properly use educational technology is not insignificant. One option is to send staff to regular technology training sessions, but many teachers will likely not continue to use skills they have learned unless they have on site support. With the recent improvements to e-training, it is also possible to train teachers externally without sending them to training sessions, saving on costs of transportation. Another option is have on staff a full time or part time technology expert whose role it is to provide on the job training for staff.

Note that, as David, Schuff and St. Louis mention, this centralization will not cut much cost off of the software licenses schools must purchase. These licenses are typically tied to either the number of devices running the software, or more often, to the number of users of the software. One way to cut a lot of costs in this area is to explore the use of open source alternatives to the expensive software packages schools usually purchase. From this author’s experience, although there are costs associated with running open source software, the free nature of the software means that these are quite often a lot less than what one would pay for proprietary software.

A typical school in the UK makes about 8,000 photocopies per student at a total yearly cost of £100,000 per school (Spencer, 2008). Given that Canadian education and UK education are not so different, we would expect to find similar costs for photocopying here as well. If a school were to use educational technology to move to paperless, they would save this cost (at least in paper) every year. The amount of time it takes to distribute electronic versions of resources to students is approximately the same as it is to distribute paper copies of resources.

With improved communication between faculty members and between students and faculty members some of the benefits are decreased costs due to fewer meetings. For example, Google Documents can be collaboratively edited and shared among staff. Time that was previously spent meeting to discuss the formation of documents, a frequent occurrence in this educator’s experience, can now be spent on more productive pursuits. For example more staff training, which is always difficult to time and pay for, can happen during what used to be meeting time.

Video conferencing has been used by many organizations as a way to save money. Cisco saved more than $100 million in travel and business expenses by switching much of their customer relations to video conferencing using their own technology (Manyika, Sprague, Yee 2009). Instead of sending educators to training sessions abroad and spending a fortune on travel expenses, schools could collaborate and create virtually cost-free webinar training sessions. Smaller schools could band together and use video conferencing to increase their course offerings, by allowing one teacher to serve more than one classroom simultaneously.

One of the areas where a lot of time is spent as a teacher is on administrative matters. Teachers have to keep track of attendance every class, record grades for every student, manage parent contacts, organize and maintain curriculum, and calculate their class budgets. There are free online tools such as School Tool which can be used to reduce the amount of time teachers spend on these tasks.

If one is careful about the use of proprietary tools, and manages the school’s technology wisely, money can be saved. In the switch from the Microsoft Outlook email system to Google’s Gmail for its 1800 employees; one construction company saw a cost savings of nearly $2 million which is a savings of nearly $1000 per employee (Lynch, 2008). The largest part of these savings was the removal of the necessity to manage their own email servers. Schools could see similar savings per student, especially not-for-profit schools for whom the Google Education accounts are free.

Similar savings can be seen elsewhere in the school. In an interview with Chris Franzen (personal communication, December 5th, 2009), a technology coordinator at a state-funded public school, he mentioned that one of the ways his school saves money is through the use of virtual field trips. Although we can all agree that a ‘live’ field trip is preferable to an electronic field trip, the costs of such trips can be quite prohibitive, especially once the cost of teacher coverage, transportation, site visits, and other miscellaneous costs are included.

"Nearly half of the high school dropouts point to boredom and lack of interest in classes as a reason for leaving school." (Kolderie, T., McDonald & Tim 2009) Increased engagement in school caused by meaningful lessons will lead to increased graduation rates which will lead to cost benefits for society. – "1 in 3 high school students in the Class of 2006 will not graduate this year ", (Kolderie & McDonald, 2009).  Another article suggests that 82% of people in prison are high school drop-outs (Christle, Jolivette, & Nelson, 2007). Over the course of a decade the cost to the US taxpayer for dropouts is $3 trillion (Alliance for Excellent Education, 2009).

Given the opportunity to use technology meaningfully in school, students are more likely to remain in school. According to Gloucestershire College representative Perry Perrot (Coughlan, 2009) the use of Facebook in the school has increased student retention rates in their courses. He suggests that this is due to a ‘positive effect on [student] motivation’ and the comfort students feel using the social networking platform. Other examples of this phenomenon are easy to find, and many schools have opened up across North America which include technology as a focus. Not surprisingly, these schools often have lower drop-out rates than their comparable neighbourhood schools.

There are several places where schools can save money on both the cost to implement technology and the cost to run the schools themselves. Schools need to be willing to rethink the ways information in the school is transmitted and shared and develop the flexibility to use new tools where necessary.

Much of the cost savings described here have been developed in the past 5 years. At our current rate of technological advance, every two years our knowledge about technology doubles. Without an expert on staff to keep the school abreast of changes and best practices in technology use, schools will quickly become obsolete. With obsolescence comes increased costs to maintain equipment and upgrade software, as well as compete with other local schools who have kept their technological skills up to date.

Predicting the future has become increasingly difficult given the rapid rate of knowledge acquisition. We cannot now predict what jobs will exist when our current high school students graduate university, but it seems likely that they will require technical expertise given current trends in job development. Without the training in technology that secondary schools offer, the cost to train our students will have to borne by either the future employers of our students, or by society.

Although there is some resistance in education to following business models, it seems clear that what many businesses are doing to save money would work in schools. As previously stated businesses are centralizing their services, standardizing their use of IT in multiple locations and developing innovative ways to use technology. Schools would do well to observe the practices businesses follow.

The factory model of education based on how businesses operate does not work because not all children are the same. Fortunately new models of education are replacing the factory model (Matusov, Rogoff, and White 1996) where the learners have much more control over their learning.

Technology definitely helps make this learner centered learning much easier as it provides tools for student collaboration and creation. Although the expense of educational technology can be great, creative school administrators and technology coordinators can still find ways to reduce operating costs using technology.

References

Ash, K. (2009), ‘Experts Debate Cost Savings of Virtual Education’, Education Week 28(25).

Chaddock,G.R.,(2006), US High School Dropout Rate: High, But How High? The Christian Science Monitor, June 21, 2006, retrieved from www.csmonitor.com/2006/0621/p03s02-ussc.html on November 18th, 2009.

Christle, C., Jolivette, K., & Nelson, C. (2007). School Characteristics Related to High School Dropout Rates. Remedial and Special Education, 28(6), 325-339.

Coughlan, S., (2009) Facebook ‘cuts studnt drop-outs’, http://news.bbc.co.uk/2/hi/uk_news/education/8299050.stm, retrieved on December 6th, 2009

Cuban, L. (1986). Teachers and Machines: The Classroom Use of Technology since the 1920s, retrieved from http://www.csun.edu/~jnk61575/sed514/docs/cuban_intro.pdf on December 5th, 2009

David, J.S., Schuff, D., St. Louis, R., (2002), Managing your IT Total Cost of Ownership, ACM 0002-0782/02/0100, retrieved from http://portal.acm.org/citation.cfm?id=502273 on December 5th, 2009

Du Vivier, E. (2008), ‘Costs and Financing in Open Schools’, Commonwealth of Learning , Commonwealth of Learning. 1055 West Hastings Suite 1200, Vancouver BC V6E 2E9, Canada. Tel: 604-775-8200; Fax: 604-775-8210; e-mail: info@col.org; Web site: http://www.col.org

Dunleavy, M.; Dexter, S. & Heinecke, W. F. (2007), ‘What Added Value Does a 1:1 Student to Laptop Ratio Bring to Technology-Supported Teaching and Learning?’, Journal of Computer Assisted Learning 23(5), 440–452.

Guhlin, M. (2007), ‘The Case for Open Source: Open Source Has Made Significant Leaps in Recent Years. What Does It Have to Offer Education?’, Technology & Learning 27(7), 16.

Hetland, L., Winner, E. (2007). Arts for Our Sake. School Arts Classes Matter More Ever – But Not for the Reasons You Might Think. The Boston Globe, retrieved from http://www.fultonschools.org/k12/Art/documents/ArtForOurSake.pdf on July 23, 2010

Kolderie, T.; McDonald & Tim (2009), ‘How Information Technology Can Enable 21st Century Schools’, Information Technology and Innovation Foundation , Information Technology and Innovation Foundation. 1101 K Street NW Suite 610, Washington, DC 20005. Tel: 202-449-1351; Fax: 202-638-4922; e-mail: mail@itif.org; Web site: http://www.itif.org

Lynch, C.G., (2008), Cost Savings Found When Microsoft Outlook Ousted for Gmail at British Construction Firm, retrieved from http://www.cio.com/article/429863/Cost_Savings_Found_When_Microsoft_Outlook_Ou sted_for_Gmail_at_British_Construction_Firm_ on December 6th, 2009

Manyika, J., Sprague, K., Yee, L., (2009) Using Technology to Improve Workforce Collaboration, retrieved from http://whatmatters.mckinseydigital.com/internet/using- technology-to-improve-workforce-collaboration on December 6th, 2009

Matusov, E., Rogoff, B., White, C., (1996). Models of Teaching and Learning: Participation in a Community of Learners, retrieved from http://ematusov.soe.udel.edu/vita/Articles/Rogoff, Matusov, White, Models of Teaching, 1996.pdf on December 6th, 2009

McIntire, T. (2006), ‘Enough to Go ‘Round? Thinking Smart about Total Cost of Ownership’, Technology & Learning 26(12), 20.

Morrison, J. (2003) U.S. Higher Education in Transition, On the Horizon, 11(1), pp. 6-10. Retrieved from http://horizon.unc.edu/courses/papers/InTransition.html

Nadeau, B. (2008), ‘Keeping VoIP from Being the Wrong Number: Digital Phones Can Save Money for Schools but There Are Many Pitfalls’, Technology & Learning 29(4), 31.

Salpeter, J. (2009), ‘Textbook Deathwatch’, Tech & Learning 30(1), 26.

Schacter, J., (1999). The Impact of Educational Technology on Student Achievement: What the Most Current Research Has to Say, retrieved from http://www.waynecountyschools.org/150820127152538360/lib/150820127152538360/im pact_on_student_achievement.pdf on December 5th, 2009

Spencer, J., (2008). Can we give every school child in the UK a Linux notebook and still save money? retrieved from http://www.computerworlduk.com/community/blogs/index.cfm?entryid=951&blogid=17 on December 7th, 2009

Ring, S. (2008). The Edutopia Poll. Edutopia, retrieved from http://www.edutopia.org/poll-financial-crisis-school-impact on July 23, 2010

Tang, A. (2008). Economic Crisis Hits Schools. Our Education, retrieved from http://blog.oured.org/2008/10/economic_crisis_hits_schools.php on July 23rd, 2010

The High Cost of High School Dropouts: What the Nation Pays for Inadequate High Schools (2007), Alliance for Excellent Education, retrieved from http://www.adlit.org/article/19706 on November 18th, 2009

Tucker, B. (2009), ‘Florida’s Online Option’, Education Next 9(3), 12–18.

Villano, M. (2006), ‘The Road to Paperless’, Campus Technology 20(3), 40–42.

Witkowsky, K. (2008), ‘Increasing Learning and Reducing Costs through Technology: The University of Alabama Story’, Change: The Magazine of Higher Learning 40(2), 32–38.

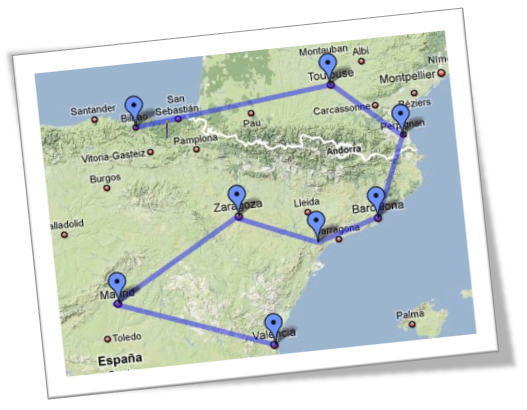I recently had students do a project where they apply the distance formula to finding the shortest path for a traveling salesperson to travel between 8 cities.  The basic idea is, the students use the longitude and latitude coordinates as substitutes for the x and y coordinates of the city, then they can use the distance formula to find a pseudo-distance between the cities.  Of course, on any kind of largish scale, this makes no sense, but on a small enough geographic scale the error in the distances is small, and I made sure the kids were aware of this deliberate error.  This project was intended to be a chance for the students to get lots of practice using the distance formula.

You can view a sample of the students work here which I have permission from the student to share.  This was rather an exceptional sample for many reasons.  The first reason is that the student went to extraordinary measures to format his document and arrange his work in a clear logical sequence.  Another reason I really enjoyed looking at this student’s project was because he had obviously done research about the traveling salesperson problem, including appropriate mathematical terminology such as the ‘Hamiltonian path’.  He talks about the limitations of the approach we took, the errors he found in the project, and suggests an improved method than the brute force solution.  He uses technological tools to his advantage, learning a bit of JavaScript to find the coordinates of the cities, and using Excel to greatly reduce his calculation time for the distances between the cities.

If all of our students work was so neatly arranged and so carefully done, I think very soon we’d soon have much different jobs.  Instead of ‘instructing our students’ we would be learning from them as equal partners.  This what I strive for in my teaching.