Tag: The Reflective Educator (page 36 of 43)

October 26, 2011 / 9 Comments

I flipped my math classroom

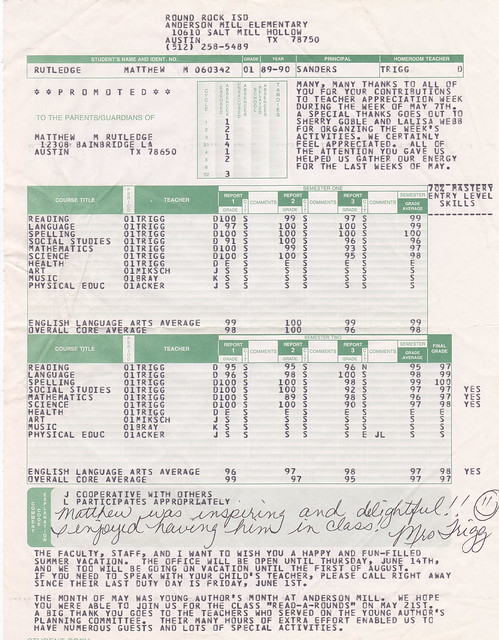
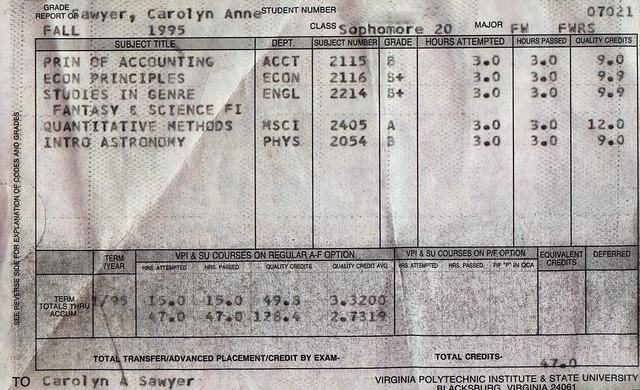
I’ve normally started my classes with a description of what math we will be learning, and a class discussion about what the math means.

When I first started teaching, I would lecture for 30 minutes, and students would work for 60 minutes (I started in with a double block of math) during double block math classes, and in a 45 minute lesson, I would still lecture for 30 minutes, and students would get 15 minutes to practice and do other activities.

I discovered early on in my teaching that the less time I talked, the more time students had to work on activities and exercises, and this led to improved understanding. I read research suggesting that adolescents could actively pay attention for about 10 – 15 minutes, so I focused on getting the lecture portion of my lesson down to this length, and on embedding more questions and subsequent discussion into my lecture.

Today I tried something new. I found questions (with an emphasis on real world application) related to exponential functions that students had never seen before, and started class by handing them out as a package, and asking students to work on these problems in groups. I then spent class circulating around the room, answering the occasional student question (but being very careful what types of questions I answered) and pushing students to try finding multiple solutions to the problems. When students were completely stuck, I offered support, but by asking them questions, rather than just giving them the solution.

Now, I’ve definitely had classes where I haven’t taught an idea to the entire class before, but this is the first time I’ve introduced a completely new topic without either presenting a lecture on the topic ahead of time or using some sort of guided instructional aid for the students (like a video prepared in advance of the lesson).

Here are some observations I had while I was circulating around the classroom.

Not one student asked me "is this solution right?"
Students were actively engaged in the problem solving process.
The questions I overheard from students (to each other) were often about the nuances in the problems, rather than "how did you do this?"
Every group of students found the most efficient standard solution to the problem, as well as 2 other ways of solving the problem.
No one attempted to Google for the solutions, or even open their textbook to see what information it had.
My students were thinking.

At the end of class, I asked students to continue working in groups and come up with notes to explain the topic. As the students will be taking an exam in about a year and half on all of the material they are writing, I recommended that they write the notes for their future self that might not remember having worked on these problems. Next class, I plan on having students form new groups, and collaborate to construct meaningful notes for the future, and then work on some more related problems.

I’ve flipped the classroom. Instead of me presenting the ideas, my students look for solutions, and I help them. Instead of me giving notes to students, they make their own notes. Instead of the classroom being about the content, it’s about the process.

There were no videos, no notes in advance, no computer assessed exercises; just a focus on changing who was doing the thinking.

October 24, 2011 / 1 Comment

It’s time to redesign the report card

The typical report card looks like this (click to embiggen):

image credit: rutlo

image credit: Richard Giles

image credit: clintjcl

A problem with these reports is they do not share with parents information that can be used to help their children improve their learning. What they share is information that is helpful to rank their children with respect to the other children in their classes. They are essentially an autotopsy of learning, rather than a document which can be used to help students improve.

A child who does "poorly" is rarely given sufficient advice to help them improve via their report card. Most comments from teachers are of the "what did David do wrong" variety, rather than "David should do x to help improve learning." A child who does well on their report card is given a free pass, and rarely pushed to extend themselves. The comments you put on your report cards should be ones that help students improve. Canned report card comments are a waste of time! If teachers do not have time to give appropriate comments for each of their students, that points to a systemic problem with classroom size (and workload) and that can’t be rectified by adding useless comments to report cards that teachers can just select.

I don’t think that online grading systems are the answer either. These lead to situations where teachers are forced into an unhealthy practice (grading everything students do) just so parents can always keep on top of the "progress" of their students. It is counter to the purpose of formative assessment to include it in an overall summative grade, and it is counter to the purpose of a summative assessment to include everything students do. Students also need a bit of freedom from their parents in order to experience this learning process themselves, and having their helicopter parents whirring around all the time checking in on them is counter-productive to them developing their own sense of independence.

We’ve used student led conferences at our school, which are an opportunity for students to show to parents directly, the results of their learning, based on portfolios our students have constructed. We are hoping to eventually have these portfolios be online, and I’d like to see the specific assessments students have done linked from their report cards. These experiences are far more valuable, both for "strong" and "weaker" students. They do have issues; not every parent takes the time to come and see their student’s work, and not every student is able to adequately explain how well they did.

I wonder what a report card that was sent home that just listed student’s (apparent) strengths and weaknesses would look like? Could you send home information that helps students improve, rather than information which helps them be numbered?

Could we design an electronic report card that gave far more information than our current ones do? What would a report card with each assessment criteria for the year, and how well our students did on each look like? Would that be too over-whelming? What if we sent home a link to an eportfolio for each student, with suggestions and comments for how to improve attached to each assessment the student does? What would happen if we gave students more ownership (perhaps with some oversight to start) over how they reported their learning to their parents?

I don’t have the answer to what the report card of the future should look like, but I do know that our current report cards need improvement.

October 23, 2011 / 4 Comments

Lessons Learned While NOT Teaching

At the keynote talk on Friday at the BCAMT conference, Dr. Peter Liljedahl shared three very interesting pieces of his research from the past ten years as an observer of classroom practice, in his lecture entitled "Lessons Learned while NOT Teaching."

Given a choice between a sitting at a desk working on paper, sitting at a desk working on a whiteboard, standing up working on a flipchart, or standing up working on a whiteboard, how would you expect students to learn best?
According to Dr. Liljedahl, students learn best while working on a non-permanent surface, while standing. He suggested that this because the non-permanence of the surface makes mistake-making easier to cope with, and that by standing up, they are on-the-stage, and so can’t hide their thinking.

How many of our classrooms are set-up to encourage this type of learning environment?
According to Dr. Liljedahl, note-taking is an activity which takes very little mental energy (from most students). Hence, if our objective is to get students to think more, we should rethink how we share notes. He recommends sharing the notes for your class after the class, and not having expectations that students will take notes from your class. Interestingly enough, this is a practice that many university professors are now moving toward as well, and it is standard practice at meetings to have minutes to share afterward. In other words, note-taking is a less useful skill today, than it might have been 20 years ago, and so it is less important that students learn the skill of taking notes. Note that I think that making notes on a topic is a different activity, and is still useful.
Is taking notes still a useful activity?
Similarly, the types of questions we answer for students has an impact on their thinking as well. From Dr. Liljedahl’s experience, students tend to ask one of three types of questions: proximity questions (which are questions asked because you are near them), stop-thinking questions (such as: "Is this right?"), and start-thinking questions (such as: "What would happen if…?"). His recommendation is to stop answering the first two types of questions. The result is that students have to spend a bit more time thinking, and that peer to peer interactions become more important.
Does this change how we ask questions?

October 23, 2011 / 2 Comments

Alan Lishness – Indigenous Innovation: How Small Places can Change the World

I’ve read about this information about the Finnish schools before, but it is nice to have it all collected into one talk. My thanks to Adam Burk for sharing it.

How does Finland do so much better than the US in education? They do everything differently.

Almost no standardized testing, well prepared teachers, huge respect for the teaching profession, professional autonomy for teachers, competitive entry system for teachers, a system controlled from the bottom up, and a much reduced emphasis on competitiveness in their education system.

October 22, 2011 / 0 Comments

Resources shared at my sessions at the BCAMT conference

Here are some of the resources I shared or mentioned during my presentations today.

Interactivity and Multimedia in Mathematics.

Dan Meyer‘s TED talk – Math class needs a makeover

Math in the Real World Flickr group

Here are the videos I shared during my presentation.

Here’s a list of free tools for math education, some of which I shared during my first presentation on Interactivity in Math.

A collection of math projects I’ve done over the years (many of these have been shared with me by colleagues).

Here’s my presentation for the day.

Social Media in Education

Educational hashtags for Twitter

8 videos to help teachers get started using Twitter

A description of the paper blogging activity we did

October 18, 2011 / 9 Comments

Should we teach the standard algorithms for arithmetic?

Just posted this comment on this article lamenting the loss of the standard algorithms in Mathematics classrooms.

Should we teach the standard algorithms for arithmetic? Absolutely, but they shouldn’t be the only algorithms kids learn.

Why exactly is the ability to add, subtract, divide and multiply large numbers so critical? It seems clear to me that these are useful skills for numbers we will encounter in our day to day lives, and that it is useful to know that algorithms exist to work with larger numbers, but your other connections seem tenuous to me at best.

You’ve argued that without practice using algorithms, students will not be able to remember them to use them later, and this I agree with. It is a basic tenet of education that spaced repetition helps students remember how to use knowledge.

The question is, what type of knowledge is critical for students to remember? Does knowing how to multiple 39835 by 2338383 or any other arbitrarily large number assist the typical person in their life? Does it even contribute to a greater understanding of advanced mathematics? Has the number of people completing advanced mathematics degrees dropped? Statistics Canada data from 2007 suggests that it has dropped very slightly (see http://www.statcan.gc.ca/pub/81-004-x/2009005/article/11050-eng.htm) but not by an alarming amount.

Regarding your achievements as a PHD in mathematics, don’t forget, the plural of anecdote is not data. You can’t generalize from your one experience to what is useful for all of society.

Understanding how to use the algorithm seems sensible to me, but I think it is even more important that people understand algorithms (emphasis on the plural) which is probably lacking in the current curriculum as it is constructed.

One problem is that all across our society, at many different age groups, we have a lack of people using any advanced mathematical thinking to solve problems. If you look at how people solve problems similar to what they learned in school, but in a different context (see Jean Lave’s work), you find that it is rare for people to use the standard algorithms they learned in life, despite the fact that the standard algorithms are much more efficient than the various algorithms people construct for themselves. This suggests that even though the standard algorithms are more efficient, they may still not be the best algorithms to teach.

It seems to me that if over the course of a lifetime, some knowledge is going to be forgotten, the skill of learning is more important than what specific knowledge is learned.

Update: I’ve had another conversation with the author of the blog post above, and it seems I’ve over-reacted a bit. We have more in common than we disagree about.

October 18, 2011 / 1 Comment

Mathematical Modelling for Real – Exploring Mathematics

This playlist has some very interesting videos from the Open University on their YouTube channel on the topic of mathematical modelling, and how it is used in different contexts.

October 18, 2011 / 1 Comment

Eric Mazur: Memorization or understanding: are we teaching the right thing?

I recommend this talk by Eric Mazur on why he switched his teaching from lecture based teaching to peer instruction based approach. It’s more than an hour long, but it really is worth it.

How does this change how we teach? How much of what students learn in our classes is actually learned? If a student can only apply the concepts they have learned to very familiar contexts, and are completely unable to apply them in different contexts, can we really say they have learned the concepts?

October 18, 2011 / 17 Comments

I tried the Khan Academy

As an experiment, I started out the beginning of this year and tried flipping my classroom, but with a slight twist: I have extra instructional time, so students were to watch the instructional videos (from the Khan Academy and IBVodcasting.com) during classroom time. We spent about 1/3 of classtime using the Khan Academy videos and exercises, about 1/3 doing problem solving activities (like what is available on www.mathpickle.com and projecteuler.net), and the rest of the time attempting to put the knowledge we were learning into a useful context for the students. While students were involved in these activities, I spent my time circulating the classroom and providing individual and small group support and instruction.

After a month I ended my experiment and am currently in a state of transition while I explore other possible ways of running my classroom. Here are some of the reasons I ended it.

Some students chose, despite repeated requests from me, to only watch videos and do exercises that were really easy for them, instead of advancing their knowledge. One student said "she liked the easy videos because it was easy to get points." Another student said she chose the easy exercises because "she was worried about getting problems wrong." These students were more focused on getting easy points and avoiding challenges than learning.
Some of my students ignored the point system of the Khan Academy and focused on learning, but found that the information from the Khan Academy wasn’t challenging enough. When given practice questions from the course content, they found that the Khan Academy style questions didn’t adequately prepare them. This was partially addressed for these students by switching to the IBVodcasting.com videos, since they are more difficult.
A few students were able to "master" the content in the Khan Academy exercises after watching a few of the videos, but were unable to transfer what they had learned to any other context, and when queried in more depth, lacked basic understand of what they were learning. For example, they could solve problems like log10 + log2 = log20, but had no idea how to find the value of log20 in terms of p and q when log10 = p and log2 = q.

I’m hoping to implement the RME model and looking for resources that will help support the course curriculum I’m required to cover in the International Baccalaureate program. If I can’t find resources to support this, I’m switching back to my style where I spend some time with students doing experiments in math, some time working on practice problems, and some time with me explaining mathematical concepts. I’m definitely not using the Khan Academy videos again (but I will probably use the IBVodcasting.com videos as additional support for students).

See this Slideshare presentation for a description of what the RME model looks like.

What is Realistic Mathematics Education? National Mathematics Conference Swakopmund

View more presentations from jdewaard

;

October 17, 2011 / 3 Comments

Should labs be part of science education?

First some misconceptions about science:

What if we create really clear explanations to address these misconceptions?

What if we run experiments with students? What if they design their own experiments to test out their hypothesis? (Really recommend watching this until the very end.)

Are there some scientific facts which are useful to know? Definitely. We could teach most those facts in a single science course if that was the purpose of science education. Why then do we teach science for 13 years in school?

A recent article suggesting that labs are "a waste of time" in science assumes that the purpose of science education is to transfer information. Instead, I believe that kids should learn that science is about experimentation and testing ideas, and that the facts which comprise scientific knowledge have been discovered through experimentation. They should know that science is not a collection of permanent facts about the world, but that instead, what is considered true in science changes. Science is more of a way of thinking about the world than a collection of isolated facts. Science is a philosophical perspective on the world wherein we recognize that through observation, experiment, and analysis, we can learn about the world.

What would you prefer? Should students know a lot of science facts, but perhaps don’t understand how those facts were derived? Or would you prefer that students understand the scientific method deeply, but might not know as much existing scientific knowledge?