Education ∪ Math ∪ Technology
First, thanks to @fnoschese for finding this video.
An Algebra Class Uses the iPad from MindShift on Vimeo.
This isn’t using technology effectively. If someone thinks this is the school of the future, and the school I send my son to decides to do this? I’d quit my job so I can homeschool him myself.
Hi all,
When I first started my career I struggled. A lot. My first job was in the School for Legal Studies which when I joined it was a relatively small high school by New York standards. I had three classes each day, two of which were double period classes. If you’ve ever watched Michelle Fiefer’s "Dangerous Minds" you’ll understand what my classes were like. It took me 3 months before I actually got one of my class’ attention.
I had one lesson which worked really well during my first semester. It was suggested to me by an Assistant Principal for Math. Basically, I started a class singing the quadratic formula song. Instantly the class went quiet. One student asked me to sing it again, so I did. By the third time I was singing it, some students were joining in. By the fifth time, only the quietest and shyest of kids weren’t singing with me. After the singing I managed to hold their attention for 20 minutes of examples of how to actually use the quadratic formula to solve equations.
For three weeks every time my students came to class, they sang the quadratic formula song when they entered. I’m still in touch with some of the students from this class and all of them remember that we sang a quadratic song although most of them don’t remember all of the words.
Over the next three years, I learned a bunch of tricks to help students memorize the bits and broken pieces that represented the NY State Math curriculum. Together my students and I sang songs to remember formulas, used hand signals to remember the relationship between an implication and its inverse, converse, and contrapositive, and deciphered calculations of algebraic groups to look for transposes and inverses. None of it made any sense to the students, it didn’t have to, they could memorize it.
I didn’t use flash cards or other tricks to help my students memorize these math facts. I used every other trick I could think of. I became a master of memorization. My students did reasonably well on their exams each year compared to their peers in other classes but I never felt like I achieved more than mediocre success because my pass rates really never exceeded 60% overall.
I regret that I did this. I wish I had more guts back then and had been willing to slow down and instead of trying to race through a bunch of disconnected concepts that I pulled out the ones which were most relevant in these students’ lives. I also wish that I had discovered my constructivist methods of teaching earlier in my career.
This actually isn’t the whole story; this is what I regret most from those early years. I also remember another side to this story which was that of an educator who endlessly experimented with different techniques to help his kids understand math.
I remember staying after school with students building model water slides so we could experiment with time-distance graphs. I remember bringing in pictures of buildings in my students’ neighbourhood so my classes could figure out the equation of the lines in the pictures. I remember buying a class set of long tape measures and protractors so we could go outside and calculate the height of the gigantic block which passed for a school in NYC. I remember being a good educator.
I do regret the endless drills and worksheets I passed out to my students. I am also eternally grateful that I found another way, a better way, and no longer rely on cheap parlor tricks in my teaching.
@joe_bower said he was looking for some decent math games online and couldn’t find any. I remembered that I used to make math games all the time so I fired off a bunch of links back. I decided it was worth gathering all those links into one spot. Who knows, maybe some of these old games are useful.
Good for teaching about perspective: Labyrinth
Gives students a feel for how fraction operations work: Fractions operations
Practice operations and solve a number puzzle: Countdown
See how simple rules result in much complexity: John Conway’s Game of Life
Practice factoring numbers & remember prime numbers: Factors!
Just a fun spaceship game (similar to Asteroids): Spaceship game
Useful for recognizing some similar fractions: Horse Races
Really just algebra practice & puzzle solving: Algebra puzzle
More of the same type as practice as above but quadratic algebra: Quadratic algebra puzzle
Look at patterns when moving rings: Towers of Hanoi
Try a variation of the classic logo programming language: Logo Programming
Here are some ideas for using sound in your classroom to help your students understand concepts through another of their senses.
There’s a fun experiment you can do with students where you bounce a ball and they watch the ball bouncing and try and measure the height of the ball as it reaches the tops of its bounce. Graph the number of the bounce versus the height of the bounce and you have an example of exponential decay. Unfortunately the results you get from students tend to look like straight lines because of the enormous potential for error in measurement.
Here’s another way to collect the data. Set up a sound recorder in your classroom. I used my iPhone but a laptop with a Mic would work. Ideally anything that can record sound in a digital format should work. Now turn on the recorder and bounce the ball close enough to the recorder that it can pick up the sound of the ball bouncing but not so close it gets damaged. Stop the recording when the ball stops bouncing. Now you open up the audio recording with an audio editor, like Audacity for example, and take a look at the recorded audio.
(listen to the sound of this ball bouncing here)
You can see from the image above that the bounces are really obvious in the recording. If you click on each bounce Audacity happily reports it’s time position in the recording to a very high level of accuracy, and to determine the amount of time between bounces, you can just subtract the time positions of any two adjacent bounces. Graph the number of the bounce versus the time until the next bounce and you’ll still get a nice exponential decay function which was the whole point of the original experiment but now your experimental error is much smaller.
Want to provide all of your students with feedback about their essays but didn’t feel like you have the time? Annoyed that all they do is check the actual grade instead of your valuable feedback?
Why not record your feedback in audio instead of writing it down? You can talk much faster than you can write and you can put the numerical grade (if you feel like you need it) into the recording itself so your students will listen to your feedback to find out what their grade. You’ll be making your feedback more useful and faster to create.
In Moodle there is a plugin which is very useful for this called Nanogong. It allows you to embed audio recordings in any of the text fields which means you can add an audio recording when you are providing feedback for your student’s online assignments.
If you have students who struggle with the written word, have them speak aloud their ideas and record the audio. They can then transcribe what they have spoken and use it in their writing. There are some useful programs for doing the transcriptions, like the Dragon Speaking Naturally app for the iPhone. They could also call a Google Voice number and leave a voice message which will be transcribed for them. In both cases there will be lots of editing work to do after they have the audio transcribed.
Besides the obvious, listening to lots of the language in many different contexts (music, radio, talk shows, etc…) have your students record themselves speaking sentences and then listen to what they sound like. Have them compare the words they are saying to what the words should sound like. Rinse and repeat. Students can then practice their pronunciation on their own without as much direct feedback from the teacher.
It also worth noting here that an actual conversation with someone in that second language is possible (and probably more desirable) through programs like Skype. Check out Around the World with 80 schools as a good place to get started connecting your classroom to the rest of the world. You may also want to see the iEARN project for global connections.
You could have students listen to a historical speech. For example you could have students listen to the actual audio from Martin Luther King Jr’s famous "I have a Dream" speech. Students might recognize that when they listen to the entire speech that his message is slightly different than the version which is highly abridged. Have students create their own "historical" speeches that might have been from different famous figures from through out history or alternatively have kids act out historical figures in a podcast play.
Have students listen to folk music from around the world through the Folkways website. They could then take their own folk music and create their own recordings and share them with their peers. Through this medium they can learn part of what the differences are between people from around the world, but more importantly our similarities.
Here’s an idea: have students record notes played on one of their musical instruments. Try and record every note from middle A to an octave higher. View the recorded notes in a tool known as an oscilloscope (try this Oscilloscope you can use on your Windows computer). Now students can actually measure the frequency of the sounds they are listening too and see a relationship between the music they like to listen to and play and that stuff about waves you were trying to teach them.
Want to teach students about the Doppler effect? How about a demonstration using a portable sound recorder, someone running around (with the recorder) and a loud sound maker of some sort, ideally something that makes a sustained pitch. Students will be able to hear the difference in the sounds as the person passes by the sound maker. This might be even better down with a video recording of the person moving timed to match the audio recording taken by the person.
Although most of these ideas involve some technology, I think that you can see that many of them can be replicated fairly easily. Want to give your students feedback about their essay? Talk to them in private. Want to connect your students to speakers of the second language they are learning? Invite them to your classroom. The point is to try and connect your students to what they are learning and to try and engage more of their senses.
I just read this article from 2007, originally posted in the Boston Globe, but available here online. The point of the article is that participation in an Arts class helps students learn skills which may not be present elsewhere in their school as a result of a narrowing focus of schools on standardized testing. To summarize the article, students can learn reflection, "such skills include visual-spatial abilities, reflection, self-criticism, and the willingness to experiment and learn from mistakes" (Hetland & Winner, 2007).
It sounds to me like this list of skills closely resembles what we would consider critical thinking skills. Certainly it is an important set of skills and if this is the only place students are learning these skills, then Arts classes are critically important. However, I know that I teach these skills in my own academic area of mathematics, and that this is possible for me because I do not have to focus on a huge standardized test at the end of the school year.
In my mathematics class students are expected to write out their solutions to problems, and to reflect on what we do. Students take turn blogging about what happened in class, and commenting on each others’ summaries. Assessment is done using projects for which students are given time to detail complete solutions, and more importantly detail the thinking the students did to arrive at these solutions. Students have to evaluate their own work, and look for ways to improve it.
We take the time to do experiments in class to verify accuracy mathematical formulas. For example, we will go out to the soccer field and use cones to create right triangles, and then compare the actual lengths of the triangles to what trigonometry and the Pythagorean theorem say the lengths should be. We talk about experimental error, and the importance in accuracy of measurements. Students whose results differ greatly from the theory go back and do it again. If no one in the class were able to achieve the theoretical results, we would revise our experiment as a class and do it again. All sorts of mathematics can be taught through experiments and I find these experiences invaluable for the learning of the students.
Fortunately at the school I work at, Arts education is not in danger. We are a small private school and our head has recently invested in our students’ learning of art by hiring a full-time learning specialist for art. However I know this is not typical of schools, more and more Arts and Music are being removed from schools because of budgetary concerns and a desire to improve students’ performance on standardized testing. There just isn’t the time to devote to the Arts in the school-wide curriculum.
You can change your own classroom so that the Arts is embedded in what you do if your school district is too short-sighted. Critical thinking skills are too important to be discarded in favor of standardization of education.
I’m very excited as this will be my first year using experiential assessments as an end of year task. Every year before this I have been required to produce a "final exam" for each of my subjects, while for the past three years at least I have known the futility of measuring students ability accurately with a single exam. The school I work at is still in the early stages of adopting experiential exams, but they have had them running for at least one year with success.
The basic idea is, the students get given a final task to complete, which is a cross-disciplinary assessment of what the students have learned how to do this year. The objective is that a few subjects get together and find a common guiding question for their assessment. Teachers from these subjects work together to create a task which can be assessed using their own criteria from each subject. We’ve chosen to break the task into pieces for each subject, but ideally there should be one complete task for the student to do.
Here are some examples, which I can finally share because the students have been introduced to the tasks themselves (and so they are no longer a secret). I have to tell you, I have been waiting to write this blog post for more than a month! Note that the students will have several hours to complete these tasks, broken up into 4 or sometimes 5 blocks of time.
In the 9th grade, our guiding question is, "How as Imperialism affected our society?" and we are looking a specific focus of Central and South America and the colonization of those parts of the world. In Mathematics, my task was, "Determine how much sugar could a galleon carry?" which was relevant because sugar is an example of a trade resource upon which the colonies depended. Here is the task sheet I provided to the students. You can see that the task is open-ended, that there is no one specific solution, and that what I will be grading the students on is the process they will be going through. The task also involves a wide variety of mathematics from the year, and I can generally assume that if the students are unsure about how to include a specific piece of mathematics, then they didn’t really get it.
This is also the kind of task that students might actually find interesting. In the creation of their diagrams to help explain themselves, there is a large amount of creative license which can be applied. When the students decide on their assumptions, which they have to justify, they can have all sorts of wild assumptions, provided there is some reasonable basis for their assumption.
Galleons are also pretty cool. They have been popularized by movies like Pirates of the Caribbean, so the students are very likely to have some personal idea of what they are like. The photo shown here is from the Wikipedia article about Galleons, and is licensed under a Creative Commons license.
This type of task also lends itself well to differentiation, as the students who wish to present more of their knowledge and understanding can take into account more factors which could affect the amount of sugar these Galleons could hold. For example, the sugar to be transported would almost certainly be done so in as water-tight barrels as the merchants could find.
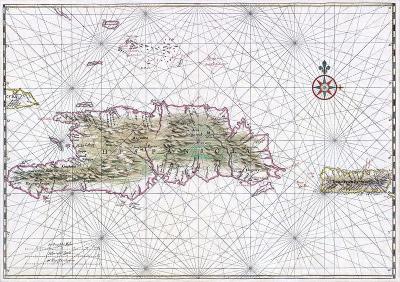In the 10th grade, our guiding question is, "How do we best get our voice heard? Is it through Science, Math, or Language?" We start by gathering evidence in all three subjects, specifically on the environmental effect of large multinational organization policies can have on small impoverished countries. We complete our week with a trial, in which students will present their scientific or mathematical evidence to their teachers. They will also role-play either French speaking or Spanish speaking people’s of said countries (we originally said that this case was a comparison of the Dominican Republic and Haiti) who have been affected by the multinational organization.
Image on the right is of the island of Hispaniola and is from a Wikipedia article about said island. It is also licensed under a Creative Commons license.
I’ve collected some data sources, through my contacts on Facebook actually, and will share these sources with the 10th grade students as a starting place. The best part is, most of the data is largely unprocessed, which means the students will have to do this themselves! In mathematics, the objective is to analyze the data and depending on whether they side with the multinational or the local population, build a case to present in the trial. Here is a copy of the task sheet we provided.
The day after the trial, students reflect on their contribution in each subject and we wrap up the trial with some conclusions. It will be really interesting to see what results.
I’m pretty pleased with the design of our experiential exams this year, and I’ll talk more about how well they went after I’ve finished this week, which looks like it will be extremely busy.
I read an article one time which questioned why we choose calculus to be the top of the math pyramid in school. Basically, most of the mathematics students learn once they master the basics aims toward preparing the students to take calculus at the end of K-12 school. The article I read suggested that statistics instead of calculus should be at the top because it is much more practical to real life than calculus is.
We deliberately choose calculus to be at the top because we want our society to produce more engineers and scientists. This helped produce a generation of engineers and scientists.
However, although engineers and scientists are still needed, the US Department of Labor predicts that neither engineers nor scientists will be in the fastest growing jobs in the future. They have predicted the 30 fasted growing jobs in the United States and there is something interesting about the list. 5 of the jobs involve the use of computers. Jobs number 25, 24, 23, 4, and 1 all include the significant use of computers in a highly technical fashion. In fact all 5 of these jobs require computer programming skills to some degree.
So I propose that we make computer programming skills should be at the top of the list. This way we will be preparing our students for careers in the future rather than the careers of the past.
Now we will still end up producing engineers and scientists because there is a huge overlap between the mathematics required to master calculus and the skills required to master computer programming. We will end up producing a lot people who are totally capable of programming a computer. Students who do not end up completing the stream will still end up having a very good understanding of how a computer works, which is obviously going to be an advantage in the future anyway.
I suspect that the current stream of math would end up diverging just after algebra. It would end up involving a lot more number theory and logical reasoning and a lot less graphing and physics based mathematics (except for the stream of students interested in game programming). I don’t know that students would find this much more interesting, but at least it would pretty easy for them to use the math they were learning and use it in direct applications involving their favorite technological devices.
Maybe kids might enjoy math more?